4 Applications: Systematic search for low-enthalpy carbon allotropes

It is well known that graphite transforms to the thermodynamically stable diamond at high pressures (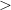 12 GPa) and high temperatures (1900-2500 K) ²³¹. On the contrary, several experiments reported that cold compression of graphite produces a metastable superhard and transparent phase, clearly different from diamond or lonsdaleite, but the exact crystal structure could not be determined ^{199; 232; 233; 234; 235}. The difficulty to experimentally resolve the crystal structure has stimulated theoretical efforts ^{4; 25; 87; 88; 236; 237; 238; 239; 240; 241; 242}. Several structural models were found using different techniques. The physical properties of these models (M-carbon ^{4; 25}, W-carbon ²³⁶, oC16 (also called Z-carbon) ^{237; 238; 239}, R/P carbon ²⁴⁰, bct-C ^{87; 88; 241}) have been intensely studied. Simulated x-ray diffraction patterns and band gaps of these models are mostly in good agreement with experimental data, making it even harder to decide which one is the metastable product observed in experiments. On the other hand, it is not guaranteed that there is not even a better solution for this experimental puzzle. Furthermore, it is likely that different metastable phases will be obtained by room-temperature compression of different polytypes of graphite, or under various non-hydrostatic conditions. This motivates us to do a systematic search for low-energy metastable carbon allotropes.

So far, there are several methods to find the ground state structures of unknown materials. However, none of them are designed to search for metastable states. Our recently proposed evolutionary metadynamics method ²⁴³ can focus on that task. Starting from a reasonable initial crystal structure, with this technique one can produce efficiently both the ground state and metastable states accessible from that initial structure. In this paper, we applied this technique to systematically search for metastable carbon allotropes accessible from graphite. Starting the calculation at 20 GPa from two polytypes of graphite (2H and 3R), we easily found the diamond structure (ground state) and a number of low-energy metastable structures with sp hybridization which could possibly explain ‘superhard graphite’.

In a compression experiment at low temperatures (low enough to preclude transition to the stable state), the product depends on the nature of the starting materials, and on the energy landscape (in particular, energy barriers). To fully investigate the possible candidate materials, we performed simulations starting from two different graphite polytypes (graphite-2H and 3R), which differ in the stacking of graphene layers.