Bibliography

[1]: J Maddox. Crystals from first principles. Nature, 335:201, 1988.
[2]: A. Gavezzotti. Are crystal structures predictable? Acc. Chem. Res., 27:309–314, 1994.
[3]: A. R. Oganov(ed). Modern Methods of Crystal Structure Prediction. WILEY-VCH, Weinheim, 2010.
[4]: A. R. Oganov and C. G. Glass. Crystal structure prediction using evolutionary algorithms: principles and applications. J. Chem. Phys., 124:244704, 2006.
[5]: J. Pannetier, J. Bassas-Alsina, J. Rodriguez-Carvajal, and V. Caignaert. Prediction of crystal structures from crystal chemistry rules by simulated annealing. Nature, 346:343–345, 1990.
[6]: J. C. Schon and M. Jansen. First step towards planning of syntheses in solid-state chemistry: Determination of promising structure candidates by global optimization. Angew. Chem. Int. Ed. Engl., 35:1286–1304, 1996.
[7]: R. Martoňák, A. Laio, and M. Parrinello. Predicting crystal structures: The parrinello-rahman method revisited. Phys. Rev. Lett., 90:075503, 2003.
[8]: D. J. Wales and J. P. K. Doye. Global optimization by basin-hopping and the lowest energy structures of lennard-jones clusters containing up to 110 atoms. J. Phys. Chem. A, 101:5111–5116, 1997.
[9]: C. M. Freeman, J. M. Newsam, S. M. Levine, and C. R. A. Catlow. Inorganic crystal structure prediction using simplified potentials and experimental unit cells: application to the polymorphs of titanium dioxide. J. Mater. Chem., 3:531–535, 1993.
[10]: S Goedecker. Minima hopping: Searching for the global minimum of the potential energy surface of complex molecular systems without invoking thermodynamics. J. Chem. Phys., 120:9911–9917, 2004.
[11]: S. Curtarolo et al. Crystal structures with data mining of quantum calculations. Phys. Rev. Lett., 91:135503, 2003.
[12]: A. R. Oganov, J. Chen, C. Gatti, Y. Ma, Y. Ma, C. W. Glass, Z. Liu, T. Yu, O. O. Kurakevych, and V. L Solozhenko. Ionic high-pressure form of elemental boron. Nature, pages 863–867, 2009.
[13]: Y. Ma, M. I. Eremets, A. R. Oganov, Y. Xie, I. Trojan, S. Medvedev, A. O. Lyakhov, M. Valle, and V. Prakapenka. Transparent dense sodium. Nature, 458:182–185, 2009.
[14]: A. R. Oganov and S. Ono. Theoretical and experimental evidence for a post-perovskite phase of mgsio3 in earth’s d" layer. Nature, 430:445–448, 2004.
[15]: Motohiko Murakami, Kei Hirose, Katsuyuki Kawamura, Nagayoshi Sata, and Yasuo Ohishi. Post-perovskite phase transition in mgsio3. Science, 304:855–858, 2004.
[16]: Taku Tsuchiya, Jun Tsuchiya, Koichiro Umemoto, and Renata M. Wentzcovitch. Phase transition in mgsio3 perovskite in the earth’s lower mantle. Earth Planet. Sci. Lett., 224:241 – 248, 2004.
[17]: B. Rousseau and N. W. Ashcroft. Observability of a projected new state of matter: a metallic superfluid. Phys. Rev. Lett., 101:046407, 2008.
[18]: W. Grochala, R. Hoffmann, J. Feng, and N. W. Ashcroft. The chemical imagination at work in very tight places. Angew. Chem. Int. Ed., 46:3620–3642, 2007.
[19]: J. Nagamatsu, N. Nakagawa, T. Muranaka, Y. Zenitani, and J. Akimitsu. Discovery of superconductivity of mgb2 with tc=39k. Nature, 410:63–64, 2001.
[20]: P. Prachi. Materials genome initiative and energy. MRS Bulletin, 36:964–966, 2011.
[21]: R. M. Martin. Electronic Structure. Cambridge University Press, Cambridge, 2004.
[22]: M. S. Woodley, D. P. Battle, D. J. Gale, and R. A. C. Catlow. The prediction of inorganic crystal structures using a genetic algorithm and energy minimisation. Phys. Chem. Chem. Phys., 1:2535–2542, 1999.
[23]: A. R. Oganov, A. O. Lyakhov, and M. Valle. How evolutionary crystal structure prediction works - and why. Acc. Chem. Res., 44:227–237, 2011.
[24]: K. Yang, W. Setyawan, S. Wang, M. B. Nardeli, and S. Curtarolo. A search model for topological insulators with high-throughput robustness descriptors. Nat. Mater., 5:623–626, 2006.
[25]: Q. Li, Y. Ma, A. R. Oganov, H. Wang, H. Wang, Y. Xu, T. Cui, H-K. Mao, and G. Zou. Superhard monoclinic polymorph of carbon. Phys. Rev. Lett., 102:175506, 2009.
[26]: C. W. Glass, A. R. Oganov, and N. Hansen. Uspex evolutionary crystal structure prediction. Comput. Phys. Comm., 175:713–720, 2006.
[27]: A. R. Oganov and C. W. Glass. Evolutionary crystal structure prediction as a tool in materials design. J. Phys.: Cond. Matter, 20:064210, 2008.
[28]: A. R. Oganov and M. Valle. How to quantify energy landscapes of solids. J. Chem. Phys., 130:104504, 2009.
[29]: G. H. Jóhannesson, T. Bligaard, A. V. Ruban, H. L. Skriver, K. W. Jacobsen, and J. K. Nørskov. Combined electronic structure and evolutionary search approach to materials design. Phys. Rev. Lett., 88:255506, 2002.
[30]: A. O. Lyakhov, A. R. Oganov, H. T. Stokes, and Q Zhu. New developments in evolutionary structure prediction algorithm uspex. Comput. Phys. Comm, 184:1172–1182, 2013.
[31]: Q. Zhu, L. Li, A. R. Oganov, and P. B. Allen. Evolutionary prediction of variable stoichiometric surface reconstructions. in preparation, 2013.
[32]: Q. Zhu, A. R. Oganov, M. A. Salvadó, P. Pertierra, and A. O. Lyakhov. Denser than diamond:ab initio search for superdense carbon allotropes. Phys. Rev. B, 83:193410, 2011.
[33]: A. O. Lyakhov and A. R. Oganov. Evolutionary search for superhard materials: Methodology and applications to forms of carbon and tio $_2$ . Phys. Rev. B, 84:092103, 2011.
[34]: F. Jensen. Computational Chemistry. Wiley, New York, 1999.
[35]: D. A. Case et al. AMBER 12. University of California, San Francisco, 2012.
[36]: B. R. Brooks, R. E. Bruccoleri, B. D. Olafson, D. J. States, S. Swaminathan, and M. Karplus. Charmm: A program for macromolecular energy, minimization, and dynamics calculations. J. Comput. Chem., 4:187–217, 1983.
[37]: K. Meier, N. Schmid, and V. W. F. Gunsteren. Interfacing the gromos (bio)molecular simulation software to quantum-chemical program packages. J. Comput. Chem., 33:2108–2117, 2012.
[38]: M. S. Daw, S. M. Foiles, and M. I. Baskes. The embedded-atom method: a review of theory and applications. Mater. Sci. Rep., 9:251 – 310, 1993.
[39]: P. Hohenberg and W. Kohn. Inhomogeneous electron gas. Phys. Rev., 136:B864–B871, 1964.
[40]: W. Kohn and L. J. Sham. Self-consistent equations including exchange and correlation effects. Phys. Rev., 140:A1133–A1138, 1965.
[41]: J. P. Perdew and A. Zunger. Self-interaction correction to density-functional approximations for many-electron systems. Phys. Rev. B, 23:5048–5079, 1981.
[42]: J. P. Perdew et al. Atoms, molecules, solids, and surfaces: Applications of the generalized gradient approximation for exchange and correlation. Phys. Rev. B, 45:13244–13249, 1992.
[43]: J. P. Perdew, K. Burke, and M. Ernzerhof. Generalized gradient approximation made simple. Phys. Rev. Lett., 77:3865–3868, 1996.
[44]: C. Lee, W. Yang, and R. G. Parr. Development of the colle-salvetti correlation-energy formula into a functional of the electron density. Phys. Rev. B, 37:785–789, 1988.
[45]: A. D. Becke. Density-functional exchange-energy approximation with correct asymptotic behavior. Phys. Rev. A, 38:3098–3100, 1988.
[46]: K. Burke. Perspective on density functional theory. J. Chem. Phys., 136:150901, 2012.
[47]: J. P. Perdew, A. Ruzsinszky, G. I. Csonka, O. A. Vydrov, G. E. Scuseria, L. A. Constantin, X. Zhou, and K. Burke. Restoring the density-gradient expansion for exchange in solids and surfaces. Phys. Rev. Lett., 100:136406, 2008.
[48]: A. D. Becke. Density-functional thermochemistry. iii. the role of exact exchange. J. Chem. Phys., 98:5648–5652, 1993.
[49]: John P. Perdew, Matthias Ernzerhof, and Kieron Burke. Rationale for mixing exact exchange with density functional approximations. J. Chem. Phys., 105:9982–9985, 1996.
[50]: A. V. Krukau, O. A. Vydrov, A. F. Izmaylov, and G. E. Scuseria. Influence of the exchange screening parameter on the performance of screened hybrid functionals. J. Chem. Phys., 125:224106, 2006.
[51]: S. Grimme. Semiempirical gga-type density functional constructed with a long-range dispersion correction. J. Comp. Chem., 27:1787–1799, 2006.
[52]: M. Dion, H. Rydberg, E. Schröder, D. C. Langreth, and B. I. Lundqvist. Van der waals density functional for general geometries. Phys. Rev. Lett., 92:246401, 2004.
[53]: G. Román-Pérez and J. M. Soler. Efficient implementation of a van der waals density functional: Application to double-wall carbon nanotubes. Phys. Rev. Lett., 103:096102, 2009.
[54]: J. Klimes, D. R. Bowler, and A. Michaelides. Van der waals density functionals applied to solids. Phys. Rev. B, 83:195131, 2011.
[55]: Vladimir I. Anisimov, Jan Zaanen, and Ole K. Andersen. Band theory and mott insulators: Hubbard U instead of stoner I. Phys. Rev. B, 44:943–954, 1991.
[56]: D. Vanderbilt. Soft self-consistent pseudopotentials in a generalized eigenvalue formalism. Phys. Rev. B, 41:7892–7895, 1990.
[57]: P. E. Blochl. Projector augmented-wave method. Phys. Rev. B, 50:17953–17979, 1994.
[58]: H. J. Monkhorst and J. D. Pack. Special points for brillouin-zone integrations. Phys. Rev. B, 13:5188–5192, 1976.
[59]: J. Behler. Neural network potential-energy surfaces in chemistry: a tool for large-scale simulations. Phys. Chem. Chem. Phys., 13:17930–17955, 2011.
[60]: M. C. Payne, M. P. Teter, D. C. Allan, T. A. Arias, and J. D. Joannopoulos. Iterative minimization techniques for ab initio total-energy calculations: molecular dynamics and conjugate gradients. Rev. Mod. Phys., 64:1045–1097, 1992.
[61]: J. D. Gale. General Utility Lattice Program, 2003.
[62]: J. D. Gale and A. L. Rohl. The general utility lattice program (gulp). Mol. Simul., 29:291–341, 2003.
[63]: S. L. Price et al. Modelling organic crystal structures using distributed multipole and polarizability-based model intermolecular potentials. Phys. Chem. Chem. Phys., 12:8478–8490, 2010.
[64]: G. Kresse and J. Furthmüller. Efficient iterative schemes for ab initio total-energy calculations using a plane-wave basis set. Phys. Rev. B, 54:11169–11186, 1996.
[65]: J. M. Soler et al. The siesta method for ab initio order-n materials simulation. J. Phys.: Cond. Matter, 84:2745, 2002.
[66]: K. Schwarz and P. Blaha. Solid state calculations using wien2k. Comput. Mater. Sci., 28:259 – 273, 2003.
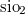[67]: A. Togo, F. Oba, and I. Tanaka. First-principles calculations of the ferroelastic transition between rutile-type and ${\text {cacl}}_{2}$ -type ${\text {sio}}_{2}$ at high pressures. Phys. Rev. B, 78:134106, 2008.
[68]: S. Baroni, S. Gironcoli, A. Corso, and P. Giannozzi. Phonons and related crystal properties from density-functional perturbation theory. Rev. Mod. Phys., 73:515–562, 2001.
[69]: S. L. Price. The computational prediction of pharmaceutical crystal structures and polymorphism. Adv. Drug Del. Rev., 56:301–319, 2004.
[70]: I. A. Baburin, S. Leoni, and G. Seifert. Enumeration of not-yet-synthesized zeolitic zinc imidazolate mof networks: A topological and dft approach. J. Phys. Chem. B, 112:9437–9443, 2008.
[71]: J. P. M. Lommerse et al. A test of crystal structure prediction of small organic molecules. Acta Cryst. B, 56:697–714, 2000.
[72]: W. D. S. Motherwell et al. Crystal structure prediction of small organic molecules: a second blind test. Acta Cryst. B, B58:647–661, 2002.
[73]: G. M. Day et al. A third blind test of crystal structure prediction. Acta Cryst., 61B:511–527, 2005.
[74]: G. M. Day et al. Significant progress in predicting the crystal structures of small organic molecules – a report on the fourth blind test. Acta Cryst., B65:107–125, 2009.
[75]: D. A. Bardwell et al. Towards crystal structure prediction of complex organic compounds – a report on the fifth blind test. Acta Cryst., B67:535–551, 2011.
[76]: S. Kim et al. Crystal structure prediction of flexible molecules using parallel genetic algorithms with a standard force field. J. Comput. Chem, 30:1973–1985, 2009.
[77]: P. Raiteri, R. Martonak, and M. Parrinello. Exploring polymorphism: the case of benzene. Angew. Chem. Int. Ed., 44:3769–3773, 2005.
[78]: G. M. Day. Current approaches to predicting molecular organic crystal structures. Cryst. Rev., 17:3–52, 2011.
[79]: A. L. Lyakhov, A. R. Oganov, and M. Valle. How to predict very large and complex crystal structures. J. Comput. Phys. Comm., 181:1623–1632, 2010.
[80]: C. P. Brock and J. D. Dunitz. Towards a grammar of crystal packing. Chem. Mater., 6:1118–1127, 1994.
[81]: W. H. Baur and D. Kassner. The perils of cc: comparing the frequencies of falsely assigned space groups with their general population. Acta Cryst., B48:356–369, 1992.
[82]: R. Hoft, J. D. Gale, and M. J. Ford. Implementation of a z-matrix approach within the siesta periodic boundary conditions code and its application to surface adsorption. Mol. Simul., 32:595–600, 2006.
[83]: Y. Li et al. Van der waals interactions in molecular assemblies from first-principles calculations. J. Phys. Chem. A, 114:1944–1952, 2010.
[84]: H. Fukazawa, S. Ikeda, and S. Mae. Incoherent inelastic neutron scattering measurements on ice xi; the proton-ordered phase of ice ih doped with koh. Chem. Phys. Lett., 282:215–218, 1998.
[85]: A. J. Leadbetter et al. The equilibrium low-structure of ice. J. Chem. Phys., 82:424–428, 1985.
[86]: B. J. Murray, D. A. Knopf, and A. K. Bertram. The formation of cubic ice under conditions relevant to earth’s atmosphere. Nature, 434:202–205, 2005.
[87]: K. Umemoto, R. M. Wentzcovitch, S. Saito, and T. Miyake. Body-centered tetragonal c4: A viable 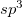$sp^3$ carbon allotrope. Phys. Rev. Lett., 104:125504, 2010.
[88]: X. F. Zhou et al. Ab initio study of the formation of transparent carbon under pressure. Phys. Rev. B, 82:134126, 2010.
[89]: J. Yang et al. ice tessellation on a hydroxylated silica surface. Phys. Rev. Lett., 92:146102, 2005.
[90]: W. B. Hubbard et al. Interior structure of neptune: comparison with uranus. Science, 253:648–651, 1991.
[91]: R. Bini and G. Pratesi. High-pressure infrared study of solid methane: Phase diagram up to 30 gpa. Phys. Rev. B, 55:14800, 1997.
[92]: H. E. Maynard-Casely et al. The distorted close-packed crystal structure of methane a. J. Chem. Phys., 133:064504, 2010.
[93]: I. Nakahata et al. Structural studies of solid methane at high pressures. Chem. Phys. Lett., 302:359–362, 1999.
[94]: L. Sun et al. X-ray diffraction studies and equation of state of methane at 202gpa. Chem. Phys. Lett., 473:72–74, 2009.
[95]: G. Gao et al. Dissociation of methane under high pressure. J. Chem. Phys., 133:144508, 2010.
[96]: A. D. Fortes et al. Ab initio simulation of ammonia monohydrate (nh3-h2o) and ammonium hydroxide (nh4oh). J. Chem. Phys., 115:7006, 2001.
[97]: F. Datchi et al. Solid ammonia at high pressure: A single-crystal x-ray diffraction study to 123gpa. Phys. Rev. B, 73:17411, 2006.
[98]: J. S. Loveday et al. Structure of deuterated ammonia iv. Phys. Rev. Lett, 76:74–77, 1996.
[99]: C. J. Pickard and R. J. Needs. Highly compressed ammonia forms an ionic crystal. Nature Mater., 7:775–779, 2008.
[100]: M. Santoro and F. A. Gorelli. High pressure solid state chemistry of carbon dioxide. Chem. Soc. Rev., 35:918–931, 2008.
[101]: B. Olinger. The compression of solid co at 296 k to 10 gpa. J. Chem. Phys., 77:6255–6258, 1982.
[102]: N. Tajima et al. First principles prediction of crystal structures of co2. Electron. J. Theor. Chem., 2:139–148, 1997.
[103]: B. Holm et al. Theoretical investigation of high pressure phases of carbon dioxide. Phy. Rev. Lett., 85:1258, 2000.
[104]: A. R. Oganov et al. Novel high-pressure structures of mgco3, caco3 and co2 and their role in the earth’s lower mantle. Earth Planet. Sci. Lett., 273:38–47, 2008.
[105]: C. S. Yoo et al. Crystal structure of carbon dioxide at high pressure: superhard polymeric carbon dioxide. Phy. Rev. Lett., 83:5527–5530, 1999.
[106]: S. A. Bonev et al. High-pressure molecular phases of solid carbon dioxide. Phy. Rev. Lett., 91:065501, 2003.
[107]: M. M. Thiery and J. M. Leger. High pressure solid phases of benzene. i. raman and x-ray studies of c6h6 at 294 k up to 25 gpa. J. Chem. Phys., 89:4255–4271, 1988.
[108]: L. Ciabini et al. High-pressure and high-temperature equation of state and phase diagram of solid benzene. Phys. Rev. B, 72:094108, 2005.
[109]: L. Ciabini et al. Triggering dynamics of the high-pressure benzene amorphization. Nat. Mater., 6:39–43, 2007.
[110]: David Van Der Spoel, Erik Lindahl, Berk Hess, Gerrit Groenhof, Alan E. Mark, and Herman J. C. Berendsen. Gromacs: Fast, flexible, and free. J. Comput. Chem., 26(16):1701–1718, 2005.
[111]: X. D. Wen, R. Hoffmann, and N. W. Ashcroft. Benzene under high pressure: a story of molecular crystals transforming to saturated networks, with a possible intermediate metallic phase. J. Am. Chem. Soc., 133:9023–9035, 2011.
[112]: J. A. Chisholm et al. An ab initio study of observed and hypothetical polymorphs of glycine. Cryst. Growth. Des., 5:1437–1442, 2005.
[113]: S. Hamad, C. E. Hughes, and C. Richard. Clustering of glycine molecules in aqueous solution studied by molecular dynamics simulation. J. Phys. Chem. B, 112:7280–7288, 2008.
[114]: A. Dawson et al. Effect of high pressure on the crystal structures of polymorphs of glycine. Cryst. Growth. Des., 5:1415–1427, 2005.
[115]: S. A. Moggach, S. Parsons, and P. A. Wood. High-pressure polymorphism in amino acids. Cryst. Rev., 14:143–184, 2008.
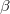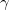[116]: V. Boldyreva, T. N. Drebushchak, and E. S. Shutova. Structural distortion of the $\alpha$ , $\beta$ and $\gamma$ polymorphs of glycine on cooling. Z. Kristallogr, 218:366–376, 2003.
[117]: G. He et al. Direct growth of 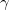$\gamma$ -glycine from neutral aqueous solutions by slow, evaporation-driven crystallization. Crys. Growth Des., 6:1746–1749, 2006.
[118]: G. L. Pervolich, L. K. Hansen, and A. Bauer-Brandl. The polymorphism of glycine. thermochemical and structural aspects. J. Thermal Anal. Calorimetry, 66:699–715, 2001.
[119]: C. Blerk and G. J. Kruger. Butane-1,4-diammonium dibromide. Acta. Cryst., E63:o342–o344, 2007.
[120]: M. Ji, C. Wang, and K. M. Ho. Comparing efficiencies of genetic and minima hopping algorithms for crystal structure prediction. Phys. Chem. Chem. Phys., 12:11617–11623, 2010.
[121]: J. L. Hoard, R. E. Hughes, and D. E. Sands. The structure of tetragonal boron. J. Am. Chem. Soc., 80:4507–4515, 1958.
[122]: M. A. Neumann and M. Perrin. The computational prediction of pharmaceutical crystal structures and polymorphism. J. Phys. Chem. B, 109:15531, 2005.
[123]: L. Schlapbach and A. Zuttel. Hydrogen-storage materials for mobile applications. Nature, 414:353–358, 2001.
[124]: B. C. Wood and N. Marzari. Dynamics and thermodynamics of a novel phase of naalh4. Phys. Rev. Lett., 103:185901, 2009.
[125]: A. Tekin, R. Caputo, and A. Züttel. First-principles determination of the ground-state structure of libh4. Phys. Rev. Lett., 104:215501, 2010.
[126]: Y. Filinchuk, B. Richter, T. R. Jensen, V. Dmitriev, D. Chernyshov, and H. Hagemann. Porous and dense magnesium borohydride frameworks: Synthesis, stability, and reversible absorption of guest species. Angew. Chem. Int. Ed., 50:11162–11166, 2011.
[127]: Q. Zhu, A. R. Oganov, C. W. Glass, and H. T. Stokes. Constrained evolutionary algorithm for structure prediction of molecular crystals: methodology and applications. Acta Cryst. B, 68:215–226, 2012.
[128]: L. George, V. Drozd, S. K. Saxena, E. G. Bardaji, and M. Fichtner. Structural phase transitions of mg(bh4)2 under pressure. J. Phys. Chem. C, 113:15087–15090, 2009.
[129]: R. Cerny, Y. Filinchuk, H. Hagemann, and K. Yvon. Magnesium borohydride: Synthesis and crystal structure. Angew. Chem. Int. Ed., 46:5765–5767, 2007.
[130]: J. Her et al. Structure of unsolvated magnesium borohydride mg(bh4)2. Acta Cryst., B63:561–568, 2007.
[131]: A. Bil, B. Kolb, R. Atkinson, D. G. Pettifor, T. Thonhauser, and A. N. Kolmogorov. van der waals interactions in the ground state of mg(bh4)2 from density functional theory. Phys. Rev. B, 83:224103, 2011.
[132]: R. Cerny, D. B. Ravnsbak, P. Schouwink, Y. Filinchuk, N. Penin, J. Teyssier, L. Smrcok, and T. R. Jensen. Potassium zinc borohydrides containing triangular [zn(bh4)3]- and tetrahedral [zn(bh4)xcl4-x]2- anions. J. Phys. Chem. C, 116:1563–1571, 2012.
[133]: V. Ozolins, E. H. Majzoub, and C. Wolverton. First-principles prediction of a ground state crystal structure of magnesium borohydride. Phys. Rev. Lett., 100:135501, 2008.
[134]: J Voss, J S Hummelshøj, Z Łodziana, and T Vegge. Structural stability and decomposition of mg(bh4)2 isomorphsan ab initio free energy study. J. Phys. Cond. Matt., 21:012203, 2009.
[135]: X. F. Zhou et al. Crystal structure and stability of magnesium borohydride from first principles. Phys. Rev. B, 79:212102, 2009.
[136]: J. Fan, K. Bao, D. F. Duan, L. C. Wang, B. B. Liu, and T. Cui. High volumetric hydrogen density phases of magnesium borohydride at high-pressure: A first-principles study. Chin. Phys. B, 21:086104, 2012.
[137]: G. Henkelman, Arnaldsson A., and H. Jonsson. A fast and robust algorithm for bader decomposition of charge density. Comput. Mater. Sci., 36:354 – 360, 2006.
[138]: F. Birch. Elasticity and constitution of the earth’s interior. J. Geophys. Res., 57:227, 1952.
[139]: H. A. Levy and P. A. Agron. The crystal and molecular structure of xenon difluoride by neutron diffraction. J. Am. Chem. Soc., 85:241–242, 1963.
[140]: D. H. Templeton, A. Zalkin, J. D. Forrester, and S. M. Williamson. Crystal and molecular structure of xenon trioxide. J. Am. Chem. Soc., 85:817–817, 1963.
[141]: S. Hoyer, T. Emmler, and K. Seppelt. The structure of xenon hexafluoride in the solid state. J. Fluorine Chem., 127:1415–1422, 2006.
[142]: M. Kim, M. Debessai, and C-S Yoo. Two- and three-dimensional extended solids and metallization of compressed xef2. Nat. Chem., 2:784–788, 2010.
[143]: M. Somayazulu, P. Dera, A. F. Goncharov, S. A. Gramsch, P. Liermann, W. Yang, Z. Liu, H-K Mao, and R. J. Hemley. Pressure-induced bonding and compound formation in xenon-hydrogen solids. Nat. Chem., 2:50–53, 2010.
[144]: D. F. Smith. Xenon trioxide. J. Am. Chem. Soc., 85:816–817, 1963.
[145]: H. Selig, H. H. Claassen, C. L. Chernick, J. G. Malm, and J. L. Huston. Xenon tetroxide: Preparation and some properties. Science, 143:1322–1323, 1964.
[146]: D. S. Brock and G. J. Schrobilgen. Synthesis of the missing oxide of xenon, xeo2, and its implications for earthâmissing xenon. J. Am. Chem. Soc., 133:6265–6269, 2011.
[147]: W. Grochala. Atypical compounds of gases, which have been called ’noble’. Chem. Soc. Rev., 36:1632–1655, 2007.
[148]: E. Anders and T. Owen. Mars and earth: Origin and abundance of volatiles. Science, 198(4316):453–465, 1977.
[149]: C Sanloup, R. J. Hemley, and H-K Mao. Evidence for xenon silicates at high pressure and temperature. Geophys. Res. Lett., 29:1883–1886, 2002.
[150]: C. Sanloup, B. C. Schmidt, E. M. C. Perez, A. Jambon, E. Gregoryanz, and M. Mezouar. Retention of xenon in quartz and earth’s missing xenon. Science, 310:1174–1177, 2005.
[151]: A. R. Oganov, Y Ma, C. W. Glass, and M Valle. Evolutionary crystal structure prediction: overview of the uspex method and some of its applications. Psi-K Newsletter, 84:142–171, 2007.
[152]: W. A. Caldwell, J. H. Nguyen, B. G. Pfrommer, F. Mauri, S. G. Louie, and R. Jeanloz. Structure, bonding, and geochemistry of xenon at high pressures. Science, 277:930–933, 1997.
[153]: R. Dovesi, V. R. Saunders, C. Roetti, R. Orlando, C. M. Zicovich-Wilson, F. Pascale, B. Civalleri, K. Doll, N. M. Harrison, I. J. Bush, Ph. D’Arco, and M. Llunell. CRYSTAL06 User’s Manual. University of Torino, 2006.
[154]: M. D. Towler, N. L. Allan, N. M. Harrison, V. R. Saunders, W. C. Mackrodt, and E. Aprà. Ab initio study of mno and nio. Phys. Rev. B, 50:5041–5054, 1994.
[155]: K. A. Peterson, D Figgen, E Goll, H Stoll, and M Dolg. Systematically convergent basis sets with relativistic pseudopotentials. ii. small-core pseudopotentials and correlation consistent basis sets for the post-d group 16?18 elements. J. Chem. Phys., 119:11113–11123, 2003.
[156]: C. Gatti. TOPOND-98: An Electron Density Topological Program for Systems Periodic in N (N=0-3) Dimensions, User’s Manual. CNR-ISTM, Milano, 1999.
[157]: C. Gatti. chapter 7, The Quantum Theory of Atoms in Molecules: From Solid State to DNA and Drug Design. Wiley-VCH, 2007.
[158]: T. Keith. Molecules in Magnetic Fields. PhD thesis, McMaster University, 1993.
[159]: M. Shishkin and G. Kresse. Self-consistent 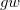$gw$ calculations for semiconductors and insulators. Phys. Rev. B, 75:235102, 2007.
[160]: V. S. Urusov. Theory of Isomorphous Miscibility. Nauka, Moscow, 1977.
[161]: Y. Ma, A. R. Oganov, and C. W. Glass. Structure of the metallic 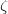$\zeta$ -phase of oxygen and isosymmetric nature of the 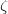$\epsilon$ - $\zeta$ phase transition: Ab initio simulations. Phys. Rev. B, 76:064101, 2007.
[162]: L. F. Lundegaard, G. Weck, M. I. McMahon, S. Desgreniers, and P. Loubeyre. Observation of an o8 molecular lattice in the [epsiv] phase of solid oxygen. Nature, 443:201–204, 2006.
[163]: D. R. Sears and H. P. Klug. Density and expansivity of solid xenon. J. Chem. Phys., 37:3002–3006, 1962.
[164]: Y. Sonnenblick, E. Alexander, Z.H. Kalman, and I.T. Steinberger. Hexagonal close packed krypton and xenon. Chem. Phys. Lett., 52:276 – 278, 1977.
[165]: A. D. Becke and K. E. Edgecombe. A simple measure of electron localization in atomic and molecular systems. J. Chem. Phys., 92:5397–5403, 1990.
[166]: R. F. W. Bader. Atoms in Molecules - A Quantum Theory. Oxford University Press, 1990.
[167]: D. J. Frost, C. Liebske, F. Langenhorst, C. A. McCammon, R. G. Tronnes, and D. C. Rubie. Experimental evidence for the existence of iron-rich metal in the earth’s lower mantle. Nature, 428:409–412, 2004.
[168]: F. W. Zhang and Oganov A. R. Valence state and spin transitions of iron in earth’s mantle silicates. Earth. Planet. Sci. Let., 249:436 – 443, 2006.
[169]: J. Lin, D. L. Heinz, H-K. Mao, R. J. Hemley, J. M. Devine, J. Li, and G. Shen. Stability of magnesiowüstite in earth’s lower mantle. Proc. Nat. Aca. Sci., 100:4405–4408, 2003.
[170]: I. I. Mazin, Y. Fei, R. Downs, and R. E. Cohen. Possible polytypism in feo at high pressures. American Mineralogist, 83:451–457, 1998.
[171]: A. R. Oganov, R. Martonak, A. Laio, P. Raiteri, and Parrinello M. Anisotropy of earth’s d” layer and stacking faults in the mgsio3 post-perovskite phase. Nature, 438:1142–1144, 2005.
[172]: V. S. Urusov and V. B. Dudnikova. The trace-component trapping effect: Experimental evidence, theoretical interpretation, and geochemical applications. Geochimica et Cosmochimica Acta, 62:1233 – 1240, 1998.
[173]: Thomas S. Duffy, Russell J. Hemley, and Ho-kwang Mao. Equation of state and shear strength at multimegabar pressures: Magnesium oxide to 227 gpa. Phys. Rev. Lett., 74:1371–1374, 1995.
[174]: M. J. Mehl, Cohen R. E., and H. Krakauer. Linearized augmented plane wave electronic structure calculations for mgo and cao. J. GeoPhys. Res., 118:8009 – 8022, 1988.
[175]: A. R. Oganov, M. J. Gillan, and G. D. Price. Ab initio lattice dynamics and structural stability of mgo. J. Chem. Phys., 118:10174–10182, 2003.
[176]: A. B. Belonoshko, S. Arapan, R. Martonak, and A. Rosengren. Mgo phase diagram from first principles in a wide pressure-temperature range. Phys. Rev. B, 81:054110, 2010.
[177]: Koichiro Umemoto, Renata M. Wentzcovitch, and Philip B. Allen. Dissociation of mgsio3 in the cores of gas giants and terrestrial exoplanets. Science, 311:983–986, 2006.
[178]: H. Wriedt. The mgo (magnesium-oxygen) system. J. Phase Equilibria, 8:227–233, 1987.
[179]: J. M. Recio and Ravindra Pandey. Ab initio study of neutral and ionized microclusters of mgo. Phys. Rev. A, 47:2075–2082, 1993.
[180]: Z.L. Wang, J. Bentley, E.A. Kenik, L.L. Horton, and R.A. McKee. In-situ formation of mgo2 thin films on mgo single-crystal surfaces at high temperatures. Surf. Sci., 273:88 – 108, 1992.
[181]: Q. Zhu, D. Y. Jung, A. R. Oganov, C. W. Glass, C. Gatti, and A. O. Lyakhov. Stability of xenon oxides at high pressures. Nature Chem., 5:61–65, 2013.
[182]: E. Zurek, R. Hoffmann, N. W. Ashcroft, A. R. Oganov, and A. O. Lyakhov. A little bit of lithium does a lot for hydrogen. Proc. Natl. Aca. Sci., 106:17640–17643, 2009.
[183]: Ross T. Howie, Olga Narygina, Christophe L. Guillaume, Shaun Evans, and Eugene Gregoryanz. High-pressure synthesis of lithium hydride. Phys. Rev. B, 86:064108, 2012.
[184]: W. Zhang, A. R. Oganov, A. F. Goncharov, Q. Zhu, S. E. Boulfelfel, A. O. Lyakhov, M. Somayazulu, and V. B. Prakapenka. Unexpected stable stoichiometries of sodium chlorides. arXiv preprint arXiv:1211.3644, 2012.
[185]: Andriy O. Lyakhov, Artem R. Oganov, Harold T. Stokes, and Qiang Zhu. New developments in evolutionary structure prediction algorithm uspex. Comp. Phys. Comm., 184:1172 – 1182, 2013.
[186]: N. Vannerberg. Progress in Inorganic Chemistry. John Wiley Sons, Inc., 2007.
[187]: S. C. Abrahams and J. Kalnajs. The formation and structure of magnesium peroxide. Acta Cryst., 7:838–842, 1954.
[188]: I. Efthimiopoulos, K. Kunc, S. Karmakar, K. Syassen, M. Hanfland, and G. Vajenine. Structural transformation and vibrational properties of bao2 at high pressures. Phys. Rev. B, 82:134125, 2010.
[189]: N. G. Vannerberg. The formation and structure of magnesium peroxide. Ark. Kemi, 14:99–105, 1959.
[190]: H. Olijnyk and W. B. Holzapfel. High-pressure structural phase transition in mg. Phys. Rev. B, 31:8412–4683, 1985.
[191]: R. M. Wentzcovitch and M. L. Cohen. Theoretical model for the hcp-bcc transition in mg. Phys. Rev. B, 37:5571–5576, 1988.
[192]: P. Li, G. Gao, Y. Wang, and Y. Ma. Crystal structures and exotic behavior of magnesium under pressure. J. Phys. Chemi. C, 114:21745–21749, 2010.
[193]: R. D. Shannon and C. T. Prewitt. Effective ionic radii in oxides and fluorides. Acta Cryst., B25:925–946, 1969.
[194]: J. T. Waber and Cromer D. T. Orbital radii of atoms and ions. J. Chem. Phys., 42:4116–4123, 1965.
[195]: Aliaksandr V. Krukau, Oleg A. Vydrov, Artur F. Izmaylov, and Gustavo E. Scuseria. Influence of the exchange screening parameter on the performance of screened hybrid functionals. J. Chem. Phys., 125:224106, 2006.
[196]: K. Tanigaki et al. Superconductivity at 33 k in cs $_ x$ rb $_ y$ c60. Nature, 352:222–223, 1991.
[197]: E. A. Ekimov et al. Superconductivity in diamond. Nature, 428:542–545, 2004.
[198]: J. C. Mayer, A. K. Geim, M. I. Katsnelson, K. S. Novoselov, T. J. Booth, and S. Roth. The structure of suspended graphene sheets. Nature, 446:60, 2007.
[199]: W-L. Mao, H-K. Mao, P. J. Eng, T. P. Trainor, M. Newville, C. Kao, D. L. Heinz, J. Shu, Y. Meng, and R. J. Hemley. Bonding changes in compressed superhard graphite. Science, 302:425–427, 2003.
[200]: Wang. Z. W. et al. A quenchable superhard carbon phase synthesized by cold compression of carbon nanotubes. Proc. Natl. Acad. Sci., 101:13699–13702, 2004.
[201]: C. J. Pickard and R. J. Needs. Hypothetical low-energy chiral framework structure of group 14 elements. Phys. Rev. B, 81:014106, 2010.
[202]: R. Hoffmann, T. Hughbanks, and M. Kertesz. Hypothetical metallic allotrope of carbon. J. Am. Chem. Soc., 105:4831–4832, 1983.
[203]: V. V. Brazhkin. Interparticle interaction in condensed media: some elements are ‘more equal than others’. Phys. Usp., 52:369, 2009.
[204]: M. D. Knudson, M. P. Desjarlais, and D. H. Dolan. Shock-wave exploration of the high-pressure phases of carbon. Science, 302:1822–1825, 2008.
[205]: R. L. Johnston and R. Hoffmann. Superdense carbon, c8: supercubane or analog of .gamma.-silicon? J. Am. Chem. Soc., 111:810–819, 1989.
[206]: R. Biswas, Richard M. Martin, R. J. Needs, and O. H. Nielsen. Stability and electronic properties of complex structures of silicon and carbon under pressure: Density-functional calculations. Phys. Rev. B, 35:9559–9568, 1987.
[207]: S. J. Clark, G. J. Ackland, and J. Crain. Theoretical stability limit of diamond at ultrahigh pressure. Phys. Rev. B, 52:15035–15038, 1995.
[208]: A. Wosylus, Y. Prots, W. Schnelle, M. Hanfland, and U. Schwarz. Crystal structure refinements of ge (tp12), physical properties and pressure-induced phase transformation ge (tp12) to ge (ti4). J. Chem. Sci, 63:608 – 614, 2008.
[209]: C. T. Prewitt and H. S. Young. Germanium and silicon disulfides: Structure and synthesis. Science, 149:535–537, 1965.
[210]: B. J. Skinner and D. E. Appleman. Melanophlogite, a cubic polymorph of silica. Am. Mineral, 48:854, 1963.
[211]: D. Bakowies, A. Gelessus, and W. Thiel. Quantum chemical study of c78 fullerene isomers. Chem. Phys. Lett., 11:324–329, 1992.
[212]: Th. Frauenheim, G. Jungnickel, Th. Kohler, and U. Stephan. Structure and electronic properties of amorphous carbon: from semimetallic to insulating behaviour. J. Non-Cryst. Solids, 182:186 – 197, 1995.
[213]: F. Gao et al. Hardness of covalent crystals. Phys. Rev. Lett., 91:015502, 2003.
[214]: F. Occelli, P. Loubeyre, and R. Letoullec. Properties of diamond under hydrostatic pressures up to 140 gpa. Nat. Mater., 2:151–154, 2003.
[215]: M. Shishkin, M. Marsman, and G. Kresse. Accurate quasiparticle spectra from self-consistent gw calculations with vertex corrections. Phys. Rev. Lett., 99:246403, 2007.
[216]: J. Paier, M. Marsman, and G. Kresse. Dielectric properties and excitons for extended systems from hybrid functionals. Phys. Rev. B, 78:121201, 2008.
[217]: M. Cardona and M. L. W. Thewalt. Isotope effects on the optical spectra of semiconductors. Rev. Mod. Phys., 77:1173–1224, 2005.
[218]: L. D. Landau, E. M. Lifshitz, and L. P. Pitaevskii. Electrodynamics of Continuous Media. Butterworth-Heinermann, Oxford, UK, 1984.
[219]: A. Laio and M. Parrinello. Escaping free-energy minima. Proc. Natl. Acad. Sci., 99:12562–12566, 2002.
[220]: R. Martonak, D. Donadio, A. R. Oganov, and M. Parrinello. Crystal structure transformations in sio2 from classical and ab initio metadynamics. Nat. Mater., 5:623–626, 2006.
[221]: M. Parrinello and A. Rahman. Crystal structure and pair potentials: A molecular-dynamics study. Phys. Rev. Lett., 45:1196–1199, 1980.
[222]: Arthur F. Voter. A method for accelerating the molecular dynamics simulation of infrequent events. J. Chem. Phys., 106:4665–4677, 1997.
[223]: K. Li, X. Wang, F. Zhang, and D. Xue. Electronegativity identification of novel superhard materials. Phys. Rev. Lett., 100:235504, 2008.
[224]: R. Martonak, A. Laio, M. Bernasconi, C. Ceriani, P. Raiteri, F. Zipoli, and M. Parrinello. Simulation of structural phase transitions by metadynamics. Z. Kristallogr., 220:489–498, 2005.
[225]: S. F. Pugh. Relations between elastic moduli and plastic properties of polycrystalline pure metals. Philos. Mag., 45:823–843, 1954.
[226]: B. W. H. Beest, G. J. Kramer, and R. A. Santen. Force fields for silicas and aluminophosphates based on ab initio calculations. Phys. Rev. Lett., 64:1955–1958, 1990.
[227]: A. R. Oganov, J. P. Brodholt, and G. D Price. Comparative study of quasiharmonic lattice dynamics, molecular dynamics and debye model in application to mgsio3 perovskite. Phys. Earth Planet. Inter., 122:277 – 288, 2000.
[228]: A. R. Oganov, G. D. Price, and J. P. Brodholt. Theoretical investigation of metastable al2sio5 polymorphs. Acta Cryst. A, 57:548–557, 2001.
[229]: V. S. Urusov, V. R. Oganov, and N. N. Eremin. Computer simulation of the structure, properties, and stability of the al2sio5 polymorphs: I. ionic model. Geochem. Int., 36:3897–414, 1998.
[230]: S. E. Boulfelfel, A. R. Oganov, and S. Leoni. Understanding the nature of “superhard graphite". Sci. Rep., 2:471, 2012.
[231]: T. Irifune et al. Materials: Ultrahard polycrystalline diamond from graphite. Nature, 421:599–600, 2003.
[232]: R. B. Aust and H. G. Drickamer. Carbon: A new crystalline phase. Science, 140:817–819, 1963.
[233]: M. Hanfland, K. Syassen, and R. Sonnenschein. Optical reflectivity of graphite under pressure. Phys. Rev. B, 40:1951–1954, 1989.
[234]: Y. Zhao and I. L. Spain. X-ray diffraction data for graphite to 20 gpa. Phys. Rev. B, 40:993–997, 1989.
[235]: W. Utsumi and T. Yagi. Light-transparent phase formed by room-temperature compression of graphite. Science, 252:1542–1544, 1991.
[236]: J. Wang, C. Chen, and Y. Kawazoe. Low-temperature phase transformation from graphite to 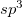$sp^{3}$ orthorhombic carbon. Phys. Rev. Lett., 106:075501, 2011.
[237]: D. Selli, I. A. Baburin, R. Martonak, and S. Leoni. Superhard sp3 carbon allotropes with odd and even ring topologies. Phys. Rev. B, 84:161411, 2011.
[238]: Z. Zhao et al. Novel superhard carbon: C-centered orthorhombic 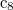${\mathrm{c}}_{8}$ . Phys. Rev. Lett., 107:215502, 2011.
[239]: M. Amsler et al. The crystal structure of cold compressed graphite. Phys. Rev. Lett., 108:065501, 2012.
[240]: H. Niu et al. Families of superhard crystalline carbon allotropes constructed via cold compression of graphite and nanotubes. Phys. Rev. Lett., 108:135501, 2012.
[241]: R. H. Baughman, A. Y. Liu, C. Cui, and P. J. Schields. A carbon phase that graphitizes at room temperature. Synthetic Metals, 86:2371 – 2374, 1997.
[242]: V. Greshnyakov and E. Belenkov. Structures of diamond-like phases. JETP, 113:86–95, 2011.
[243]: Q. Zhu, A. R. Oganov, and A. O. Lyakhov. Evolutionary metadynamics: a novel method to predict crystal structures. CrystEngComm, 14:3596–3601, 2012.