General Caption: Big Bang nucleosynthesis (cosmic time ∼ 10--1200 s ≅ 0.17--20 m) is a key element of Big Bang cosmology. The fact that calculated Big Bang nucleosynthesis is in agreement with observation (except to a degree for the cosmological lithium problem) is a key verification of said Big Bang cosmology (see below Image 6).

The situation is also reciprocal in that other verified elements of Big Bang cosmology lead us to believe calculated Big Bang nucleosynthesis is right. In fact, all well established grand theories or paradigms (as Big Bang cosmology is) are based on a network of mutually supporting verifications which gives them strong credibility.

Features:

The Big Bang nucleosynthesis era (cosmic time ∼ 10--1200 s ≅ 0.17--20 m) began at ∼ 10 s after the fiducial cosmic time of the Friedmann equation (FE) models. At this cosmic time, the cosmic temperature had fallen sufficiently low that protons and neutrons were NO longer being created by pair creation by photons (since the photons NO have the energy as the cosmic temperature falls to create such massive particles) and nuclei were NO longer being destroyed by photodisintegration (since the photons NO have the energy as the cosmic temperature falls to destroy nuclei). Also by some asymmetry in the early part of early universe (cosmic time (10**(-12) s -- 377700(3200) y), the bulk antimatter had vanished (Wikipedia: Antimatter: Origin and asymmetry; Wikipedia: Baryogenesis). So there was NO longer antimatter to complicate Big Bang nucleosynthesis.
Big Bang nucleosynthesis is the nucleosynthesis (i.e., creation) of the light elements and isotopes thereof (i.e., hydrogen (H), deuterium (D, H-2), helium-3 (He-3), Helium-4 (He-4), lithium-6 (Li-6) (very little of this), lithium-7 (Li-7)) of the primordial cosmic composition (which to a large degree is the cosmic composition) of the observable universe. To be more precise, Big Bang nucleosynthesis produced overwhelmingly most of the modern cosmic abundances of hydrogen, deuterium (of which the nuclei are called deuterons), and helium, and some significant part or maybe almost all of the cosmic abundance of lithium.
Big Bang nucleosynthesis (cosmic time ∼ 10--1200 s ≅ 0.17--20 m) occurred ∼ 13.8 Gyr ago (see Wikipedia: Age of the universe; age of the observable universe = 13.797(23) Gyr (Planck 2018) (see Planck 2018: Age of the observable universe = 13.797(23) Gyr) as measured from the probably unreal Big Bang singularity which is the formal cosmic time zero (i.e., t=0)) and, of course, the formal time zero of the Λ-CDM model (the current standard model of cosmology (SMC, i.e., the Λ-CDM model)).
Image 1 Caption: The nuclear reaction network of Big Bang nucleosynthesis.
For a somewhat more detailed image of the nuclear reaction network of Big Bang nucleosynthesis, see Hyperphysics: Big Bang Nucleosynthesis.
Image 1 Features::
1. For Big Bang nucleosynthesis, the relevant elements, nuclei, isotopes, and particles are the:
  1. gamma ray (γ): a high-energy photon.
  2. neutron (n: free n decays to p, half-life = 610(10)s with uncertainty annoyingly large): a radioactive nuclide (i.e., unstable to spontaeous radioactive decay) when a free particle with half-life 610.(10) seconds = 10.17 minutes. The free neutron decay process is
```
       n → p + e**(-) + ν**(bar)  , 
```
    where e = electron (AKA negative beta particle) and ν**(bar) = antielectron neutrino.
  3. proton (p, H): A hydrogen ion (H**(+)): (stable isotope).
  4. deuteron (D or H-2): A stable isotope) which is the atomic nucleus of the species deuterium.
  5. triton (T or H-3: decays to He-3, half-life = 4500(8) days = 12.32(2) Jyr): The atomic nucleus of the species tritium. It is a radioactive isotope with half-life 4500(8) days = 12.32(2) Julian years and radioactive decay product Helium (He-3) (see Wikipedia: Tritium: Decay). The half-life is so long compared to the Big Bang nucleosynthesis era (cosmic time ∼ 10--1200 s ≅ 0.17--20 m) that the triton is effectively a stable isotope relative to the Big Bang nucleosynthesis era (cosmic time ∼ 10--1200 s ≅ 0.17--20 m). However, all the primordial tritrium obviously decayed away rapidly in cosmic time and it made ∼ 10 % contribution to primoridial Helium (He-3) abundance (see Image 5 below) which is nearly the modern abundance.
  6. helium-3 (He-3): A stable isotope.
  7. helium-4 (He-4): A stable isotope which is much stabler than helium-3 (He-3) which is essentially why it is much more abundant than helium-3 (He-3).
  8. lithium-6 (Li-6): A stable isotope. Li-6 is very minor product of Big Bang nucleosynthesis, and so its reactions are NOT shown in the nuclear reaction network in Image 1. Big Bang nucleosynthesis predicts only ∼ 2/10**5 of lithium (Li) atoms should be a Li-6 atom (see Johnson 2014, "Big Bang ruled out as origin of lithium-6").
  9. lithium-7 (Li-7): A stable isotope.
  10. beryllium-7 (Be-7: decays to Li-7, half-life = 53.22(6) days): A radioactive isotope with half-life 53.22(6) days and has radioactive decay product lithium-7 (Li-7) (see Wikipedia: Isotopes of Beryllium: Table). The half-life is so long compared to the Big Bang nucleosynthesis era (cosmic time ∼ 10--1200 s ≅ 0.17--20 m) that Be-7 is effectively a stable isotope relative to the Big Bang nucleosynthesis era (cosmic time ∼ 10--1200 s ≅ 0.17--20 m). However, all the primordial Be-7 obviously decayed away rapidly in cosmic time and made >∼ 50 % contribution to the primordial lithium-7 (Li-7) abundance (see Image 5 below) which is a major component of the modern abundance of lithium.
2. The arrows indicate the nuclear reaction connecting ONE boxed reactant to ONE boxed product with the other reactant/product being the first/second quantity in the brackets: e.g., p(n,γ)D is the same as p + n → D + γ. The n → p is actually n → p + e**(-) + ν**(bar). The e**(-) (electron) and ν**(bar) (electron antineutrino) are omitted since they just occur as products and do NOT directly affect nuclear reaction network again.
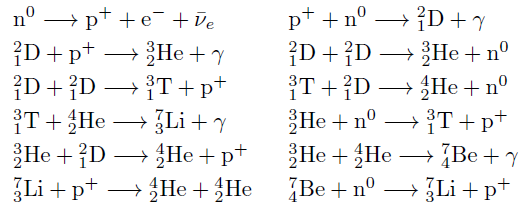
Image 2 Caption: A table of the nuclear reactions important in Big Bang nucleosynthesis. The table is somewhate more complete than the nuclear reaction network displayed in Image 1.
A key feature of this table is the reaction that is absent: the proton-proton (p-p) reaction:
```
  p**(+) + p**(+) → D + e**(+) + ν_e 
                      + 1.442 MeV  , 
```
where ν_e is electron neutrino (see Wikipedia: Proton-proton chain reaction: The proton-proton chain reaction). This reaction is many orders of magnitude slower??? than any of the shown nuclear reactions and is negligible in Big Bang nucleosynthesis. The essential reason is that an intermediate step is the formation of He-2 (diproton) which is extremely unstable and causes the overall nuclear reaction to have an extremely small cross section. When He-2 (diproton) does form successfully???, it almost immediately??? undergoes beta plus decay to complete the proton-proton (p-p) reaction. In fact, the weak nuclear force is needed to initiate the reaction and that interaction is much weaker than the strong nuclear force. (Note, the above discussion needs improvement, but that requires an improved reference.???)
In the Sun, the time-scale for the proton-proton (p-p) reaction is 7.9*10**9 yr, whereas the p + D → He-3 reaction has time scale 1.4 s (see, e.g., Ian Howarth, 2010, Astrophysical Processes: From Nebulae to Stars, Part 5, Stars II, p. 122). However, proton-proton (p-p) reaction is the initial step---and therefore the rate-determining step---in all 3 branches of the proton-proton chain (PP chain) (i.e., the pp I branch, the pp II branch, and the pp III branch) for energy generation in the Sun. All of stellar evolution is heavily dependent on proton-proton (p-p) reaction, whereas Big Bang nucleosynthesis not at all.
Note, the deuterons (D,H-2) in the Sun are all produced in the PP chain since primordial deuterons (D,H-2) were all destroyed very early in the Sun's main sequence lifetime or before by the p + D → He-3 reaction.
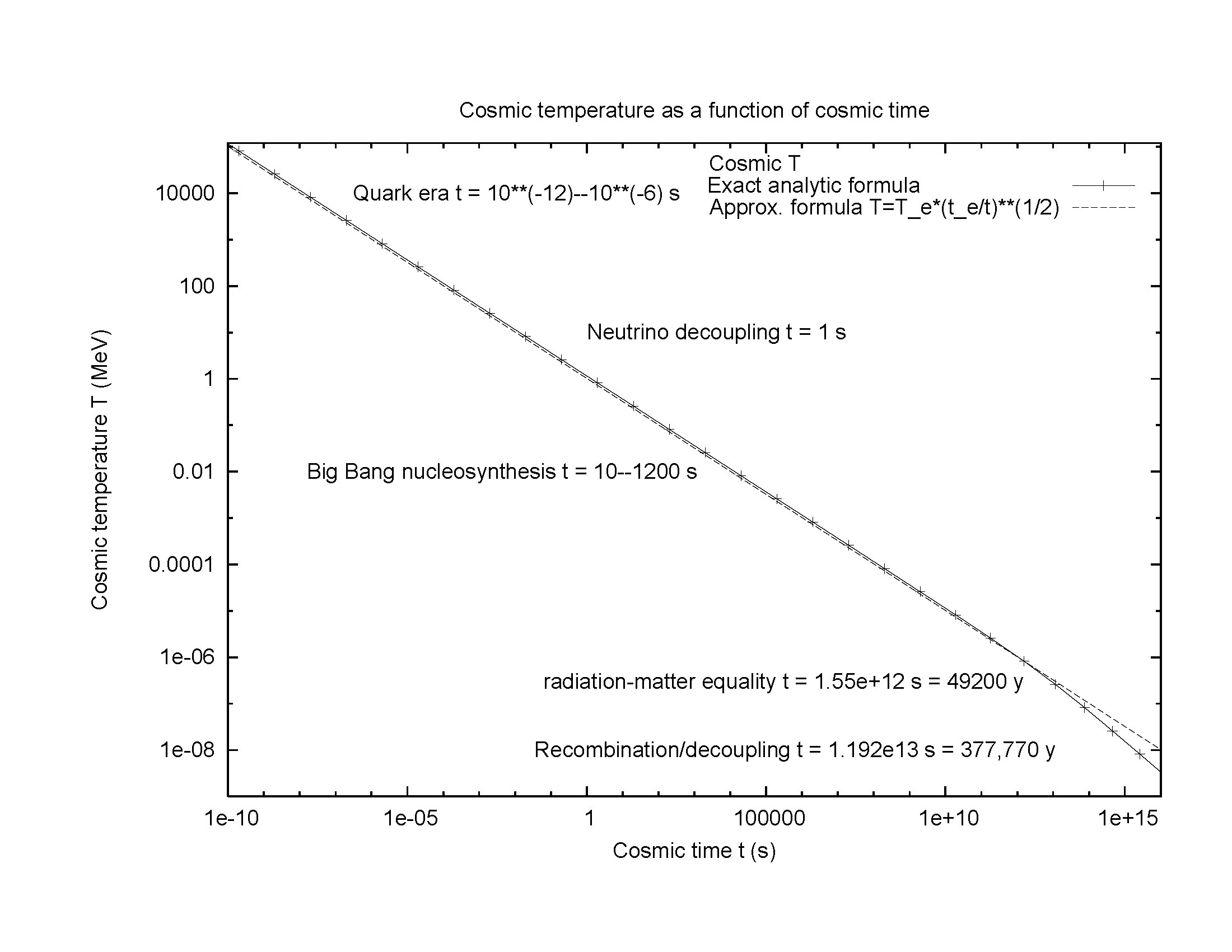
Image 3 Caption: A log-log plot cosmic temperature (in MeV ≅ 10**10 K) versus cosmic time from the quark era (cosmic time t∼ 10**(-12) -- 10**(-6) s) to the recombination era t = 377,770(3200) y (when the primordial photons stopped interacting strongly with matter).
Features of Image 3:
1. Time zero is the Big Bang singularity which probably did NOT happen. Our best theory is that the inflation era (10**(-36) -- 10**(-32) s) happened in the very early universe (t < 10**(-12) s) and then the universe tracked into a standard Friedmann-equation model thereafter.
2. The cosmic temperature is the general temperature of the observable universe. Before the matter decoupling era (cosmic time ∼ 12 Myr) (when matter stopped interacting strongly with the primordial photons), it was the temperature of all mass-energy and after that just of the primordial photons (i.e., conventionally the CMB even when NOT redshifted to the microwave band: fiducial range 0.1--100 cm) The primordial photons cooled (via the cosmological redshift and the decreasing density of photons both due to in the expanding universe) to create the cosmic microwave background (CMB) (in the microwave band (fiducial range 0.1--100 cm, 0.01--10 cm**(-1)) of cosmic present = to the age of the observable universe = 13.797(23) Gyr (Planck 2018)
3. The primordial photons has undergone cooling since the quark era at least. Before that we can only extrapolate its behavior.
4. The cosmic temperature is completely dominated by relativistic particles (as far as we know) which means that T ∝ 1/a(t), where a(t) is the cosmic scale factor. The cosmic scale factor scales as t**(1/2) before the radiation-matter equality (cosmic time t∼ 50,000). The radiation-matter equality is the transition time from the radiation era (where the observable universe's mass-energy is dominated by primordial photons) to the matter era (where the observable universe's mass-energy is dominated by matter which includes both baryonic matter and dark matter). After the radiation-matter equality , the cosmic scale factor scales as t**(2/3) thereafter. Thus, T ∼∝ 1/t**(1/2) before the radiation-matter equality (t≅ 50,000) and T ∼∝ 1/t**(2/3) thereafter. The two behaviors give straight lines on the log-log plot with slopes of, respectively, -1/2 and -2/3.
5. The displayed cosmic eras in Image 3:
  Note, time zero is the time of the probably unreal Big Bang singularity of Λ-CDM model. But though probably unreal, it is a fiducial time zero when running backward the clock of cosmic time.
Image 4 Caption: A log-log plot of the evolution with Image 4 Features:
1. Keywords for Image 4: baryons, baryon-to-photon ratio η=6.16*10**(-10) (Planck-2018) ≅ 2.75*10**(-8) Ω_b*h**2 (see also Wikipedia: Big Bang nucleosynthesis: Characteristics: baryon-to-photon ratio η = 6*10**(-10)), beryllium-7 (Be-7: decays to Li-7, half-life = 53.22(6) days), cosmic background radiation temperature (AKA temperature of the universe, lower axis), cosmic time (upper axis), deuteron (D or d, H-2), helium-3 (He-3), helium-4 (He-4), hydrogen (H or p), lithium-6 (Li-6), lithium-7 (Li-7), mass fraction (related to number abundance), neutron (n: free n decays to p, half-life = 610(10)s with uncertainty annoyingly large), proton (p, H), triton (T or t,H-3: decays to He-3, half-life = 12.32 Jyr).
2. As illustrated in the Image 4, Big Bang nucleosynthesis essentially spanned cosmic time ∼ 10--1200 s ≅ 0.17--20 m. Then it was all over.
3. The primordial cosmic composition that results form Big Bang nucleosynthesis consists of just hydrogen (i.e., protons), deuterons, helium, and a little lithium.
  Note, the primordial tritium (T, H-3) and beryllium-7 (Be-7) decayed away rapidly and conbributed to the modern abundances of, respectively, helium-3 (He-3) and lithium-7 (Li-7).
4. Note, post-main-sequence stars only contribute a small amount of helium-4 (He-4) to the modern cosmic composition compared to Big Bang nucleosynthesis (BBN).
There is a key difference between the nuclear reaction network of Big Bang nucleosynthesis and that of stellar nucleosynthesis: in Big Bang nucleosynthesis, there are free neutrons participating in the nuclear reactions.
Since neutrons are neutral, they have no Coulomb barrier (i.e., electrostatic force) to overcome to get close enough to other nuclei (which are all electrically charged) in order to undergo a nuclear reaction. The upshot is much faster nucleosynthesis is possible than otherwise such as in hydrogen burning in main-sequence stars. Of course, fast, runaway nuclear burning can happen without free neutrons (e.g., in supernovae), but other special conditions are involved.

Based on the website Thespectrum: Big Bang Nucleosynthesis (since I can't find a better source to spit it out right now), the main nucleosynthesis path in shown nuclear reaction network is probably

          p + n → D        no Coulomb barrier, but D is only weakly stable and so photodisintegration 
                                 creates the deuterium bottleneck.
                                 Temperature has to fall low enough to allow deuterium (D, H-2) to
                                 survive long enough for further nuclear reactions.
          D + n → H-3      no Coulomb barrier.
          T + D → He-4     Coulomb barrier,
                                 but the smallest one possible:  just 2 positive 
                                 elementary charges 
                                 repelling:  i.e., p and p.

Further nucleosynthesis beyond He-4 CANNOT go by just adding neutrons since the He-4 + n → products and Li-5 + n → products CANNOT survive for further nuclear reactions since He-5 (half-life = 700(30)*10**(-24) s) and Li-5 (half-life = 370(30)*10**(-24) s) are very unstable.

This bottleneck (beyond the deuterium bottleneck) brings nucleosynthesis to heavier nuclei almost to a stop.

Just a little lithium-7 (Li-7). and beryllium-7 (Be-7) get synthesized---and the latter decays away rapidly as discussed above.

Image 5 Caption: Key differences between Big Bang nucleosynthesis from stellar nucleosynthesis illustrated.

Further explication of key differences:
1. Big Bang nucleosynthesis era (cosmic time ∼ 10--1200 s ≅ 0.17--20 m): This is much shorter than the millions of years to billions of years of stellar nucleosynthesis.
2. There is an enormous distinction in that Big Bang nucleosynthesis has free neutrons (as discussed above) and stellar nucleosynthesis does NOT.
3. The Big Bang nucleosynthesis temperature is ∼ 10**9 ≅ 0.1 MeV whereas stellar nucleosynthesis has a temperature range ∼ 10**7 to 10**10 K (Google AI question: Stellar nucleosynthesis temperature range?; Wikipedia: Stellar nucleosynthesis).
4. Another difference of Big Bang nucleosynthesis from stellar nucleosynthesis (omitted in Image 5) is that heat energy feedback from the nuclear reactions in Big Bang nucleosynthesis is NOT important in Big Bang nucleosynthesis. The universal expansion of the cosmic photon gas (AKA cosmic background radiation) controls the cosmic background radiation temperature. In stellar nucleosynthesis, the heat energy from nuclear burning is a key ingredient in setting temperature.
Recall, Big Bang nucleosynthesis produced overwhelmingly most of the cosmic abundances of hydrogen, deuterons, and helium, and some significant part of the cosmic abundance of lithium-7. Image 6 below illustrated the yield of these elements.
Image 6 Caption: "This Schramm diagram depicts the predicted primordial cosmic composition of helium-4 (He-4) (purple line), deuterium (D, H-2) (blue line), helium-3 (He-3) (red line), and lithium-7 (Li-7) (green line), as a function of baryon-to-photon ratio η=6.16*10**(-10) (Planck-2018) ≅ 2.75*10**(-8) Ω_b*h**2 (see also Wikipedia: Big Bang nucleosynthesis: Characteristics: baryon-to-photon ratio η = 6*10**(-10)) on the bottom axis and equivalently baryon density parameter Ω_b*h**2 on the top axis." (Slightly edited.)
To explicate Image 6:
1. The expression Schramm diagram is in honor of David Schramm (1945--1997), one of the pioneers of Big Bang nucleosynthesis (BBN). Yours truly met Dave Schramm long ago.
2. The yellow show the observational constraints on the abundances of the primordial cosmic composition (fiducial values by mass fraction: 0.75 H, 0.25 He-4, 0.001 D, 0.0001 He-3, 10**(-9) Li-7). There is NO constraint for helium-3 (He-3) because it CANNOT yet be distinguished observationally from the much more abundant helium-4 (He-4). The nuclei of these two species are very different in behavior, but their behavior as atoms in chemistry and spectroscopy are almost identical. And remember, we know what the observable universe is made of principally from spectroscopy.
  But what you say about hydrogen (H) and deuterium (D, H-2) which are also distinct nuclei but nearly identical in their behavior as atoms? It turns out that they distinct enough as atoms for spectroscopy to tell them apart.
3. The baryon-to-photon ratio η is a free parameter for Big Bang nucleosynthesis (BBN).
4. In fact, we know the photon abundance in Big Bang nucleosynthesis era (cosmic time ∼ 10--1200 s ≅ 0.17--20 m) since that is almost constant from that time to cosmic present = to the age of the observable universe = 13.797(23) Gyr (Planck 2018). The photons in question are NOT all photons, but just those in the cosmic microwave background (CMB, T = 2.72548(57) K (Fixsen 2009)) whose abundance we can directly measure. Why is the CMB photon abundance conserved to high accuracy? The short answer is the conditions of the observable universe and thermodynamics require it.
5. However, the abundance of baryons is also conserved from the Big Bang nucleosynthesis era (cosmic time ∼ 10--1200 s ≅ 0.17--20 m), we CANNOT directly measure it to sufficient accuracy. Thus, baryon-to-photon ratio η CANNOT be known to sufficient accuracy and must be treated as aforesaid as a free parameter.
6. The baryon-to-photon ratio η=6.16*10**(-10) (Planck-2018) ≅ 2.75*10**(-8) Ω_b*h**2 show with an uncertainty fits the observations for helium-4 (He-4) and deuterium (D, H-2) extremely well.
  However, the observed lithium-7 (Li-7) is ∼ 1/3 to low for the fit. The discrepancy is cosmological lithium problem. The fact is that lithium-7 (Li-7) is both created and destroyed in stars and the common belief is that the cosmological lithium problem will be solved by better stellar nucleosynthesis calculations. But a key point is that despite the discrepancy, the agreement is between observation and prediction is still order of magnitude good.
  Overall conclusion for primordial cosmic composition (fiducial values by mass fraction: 0.75 H, 0.25 He-4, 0.001 D, 0.0001 He-3, 10**(-9) Li-7) is that there is excellent agreement over 8 orders of magnitude. Thus, there is strong evidence that we understand BBN.
7. The strong evidence for BBN gives strong faith in the fitted baryon abundance.
  However, this means that dark matter CANNOT be baryonic matter. In fact, the baryon fraction (ratio of baryonic matter to baryonic matter plus dark matter) is ∼ 1/6 = 16 % for observable universe (Ci-27) as we know from galaxy rotation curves and other evidence (see Galaxies file: galaxy_rotation.html; Galaxies file: galaxy_rotation_curve_cartoon.html).
  Besides being ruled out as as baryonic matter by BBN, dark matter is also ruled out nearly by being very, very dark. It is believed that if dark matter was baryonic matter AND as abundant as it is, then it would emit electromagnetic radiation (EMR) that is obviously coming from baryonic matter. Many theories predict dark matter does produce some EMR, but NOT nearly as much as the same amount of baryonic matter.
8. The predictions of primordial cosmic composition (fiducial values by mass fraction: 0.75 H, 0.25 He-4, 0.001 D, 0.0001 He-3, 10**(-9) Li-7) from Big Bang nucleosynthesis are compared to observations below in Table: Table: Big Bang Nucleosynthesis (BBN) Predictions and Observed Primordial Cosmic Composition
```
Table:  Table:  Big Bang Nucleosynthesis (BBN) Predictions and Observed Primordial Cosmic Composition
_____________________________________________________________________________

Element        BBN           Observed           Quantity
_____________________________________________________________________________
He (He-3,4)    0.246         0.245±0.001        mass fraction
D (H-2)        2.5           2.5 to 3           D/H, x*10**(-5)
He-3           1             none available     He-3/H, x*10**(-5)
Li-7           4.5           1.5±0.5            Li-7/H, x*10**(-10)
_____________________________________________________________________________ 
```
  Notes:
  1. The values shown are representative. Different research groups are always producing slightly updated, slightly different values.
  2. An accurate/precise? D (H-2) abundance D/H = 2.62(0.05) * 10**(-5) was reported by S. Riemer-Soerensen et al. (2017): A precise deuterium abundance: Re-measurement of the z=3.572 absorption system towards the quasar PKS1937-101.
  3. Helium (He-3) and helium-4 (He-4) CANNOT easily be distinguished observationally. They are chemically and spectroscopically nearly identical since they are both isotopes of helium. So no cosmic He-3 abundance is/was available.
  4. General reference: Mathews, G. J., et al. 2005, Phys. Rev. D, astro-ph/0408523: Big Bang Nucleosynthesis with a New Neutron Lifetime.
The anthropic principle aspect of Big Bang nucleosynthesis (BBN):
If the strong nuclear force were just a bit stronger than it is, the Big Bang would have nuclearly burned all the hydrogen into helium (see Wikipedia: Anthropic principle: Anthropic observations).
Without hydrogen, there would be NO water and NO hydrocarbons, and therefore would be NO life as we know it.
Life as we know it uses liquid water as the medium for all its chemical reactions and there is NO substitute that we think likely.
Also long-lived stars are probably needed for life as we know it and probably could NOT exist without forming as mainly hydrogen.
The upshot is that the existence of hydrogen constrains the strong nuclear force to be NOT much stronger than it is.
This upshot is an anthropic principle argument for the multiverse paradigm since there is NO known fundamental (and human-independent) reason making the strong nuclear force just as strong as it is. The strong nuclear force strength was somehow randomly chosen in different pocket universes in the multiverse paradigm and its strength in our pocket universe is below the upper bound needed for hydrogen to exist or we would NOT exist in our pocket universe---it would NOT be ours.

Images:

Credit/Permission: © User:Pamputt, 2019 / CC BY-SA 4.0.
Image link: Wikimedia Commons: File:Main_nuclear_reaction_chains_for_Big_Bang_nucleosynthesis.svg.
Credit/Permission: User:Cmbant, 2011 / Public domain.
Image link: Wikimedia Commons: File:Primordial_nucleosynthesis2.png.
Credit/Permission: © Chris Mihos, before or circa 2016 / None.
Image link: nucleosynth_fig.jpg.
Image link: Placeholder image alien_click_to_see_image.html.
The Li-6 in nucleosynth_fig.jpg looks way too high since accurate Big Bang nucleosynthesis predicts only ∼ 2/10**5 of lithium (Li) atoms should be Li-6 atoms (see Johnson 2014, " Big Bang ruled out as origin of lithium-6"). Creators of this particular Big Bang nucleosynthesis plot may have articifially increased the cross section of Li-6 to match the observed abundance in modern observable universe. The discrepancy between the Li-6 abundance from (accurate) Big Bang nucleosynthesis of observed abundance is part of the cosmological lithium problem which we discussed above.
Credit/Permission: © Tsung-Han Yeh, Keith A. Olive (1956--), Brian D. Fields 2023 (uploaded to Wikimedia Commons by User:Pamputt, 2023) / Creative Commons CC BY-SA 4.0.
Image link: Wikimedia Commons: File:Universe-09-00183-g004.png.
See also Credit/Permission: © Chris Mihos, before or circa 2016 / None.
Image link: nucleosynth_fig.jpg.
Credit/Permission: © David Jeffery, 2023 / Own work.
Image link: Itself.
Former image: Credit/Permission: © Ann Zabludoff???, before or circa 2012 / None. The now dead link Atropos: Primordial Nucleosynthesis versus Stellar Nucleosynthesis is very enlightening, but it completely omits the enormous distinction that Big Bang nucleosynthesis has free neutrons (as discussed above) and stellar nucleosynthesis does NOT.
Credit/Permission: © User:Paleo2, 2020 / CC BY-SA 4.0.
Image link: Wikimedia Commons: File:Schramm plot BBN review 2019.png.

local link: big_bang_nucleosynthesis.html

Cosmology file

big_bang_nucleosynthesis.html