General Caption: Some features of Babylonian mathematics.

1. Image 1 Caption: The Babylonian mathematicians (and their predecessors in ancient Mesopotamia the Sumerian mathematician) used a sexagesimal system (i.e., base-60 system) for mathematics and astronomy.

   In fact, the Babylonian mathematicians used the sexagesimal system consistently ONLY for mathematics and astronomy (Ne-17). In other contexts, they used other base systems. In fact, they usually used the ubiquitous decimal system (i.e., base-10 system). The decimal system is common: everyone counts on their fingers. Using a vigesimal system (i.e., base-20 system) may mean you are down to counting on your toes.

   

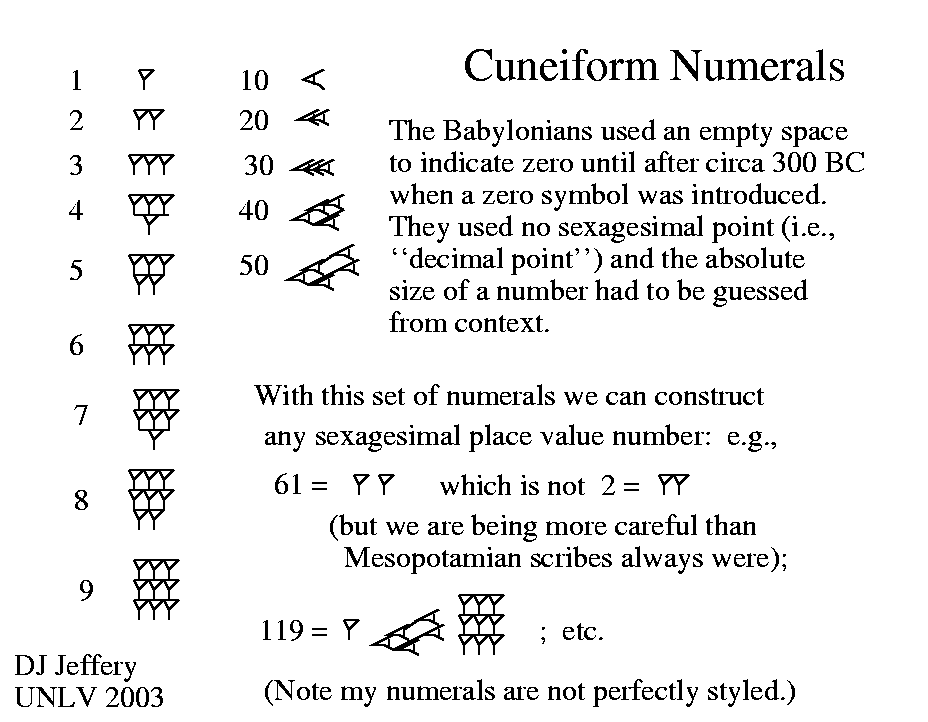
2. Image 2 Caption: The Babylonian numerals of the Babylonian sexagesimal system.

   The styling of the numerals is NOT be perfect since it is just yours truly's own handiwork.

   Note that the Babylonian mathematicians did NOT have a decimal point. Thus, the absolute size of a number had to be told from context.

   For example, without a decimal point 301 could be three hundred and 1 or thirty and one tenth.

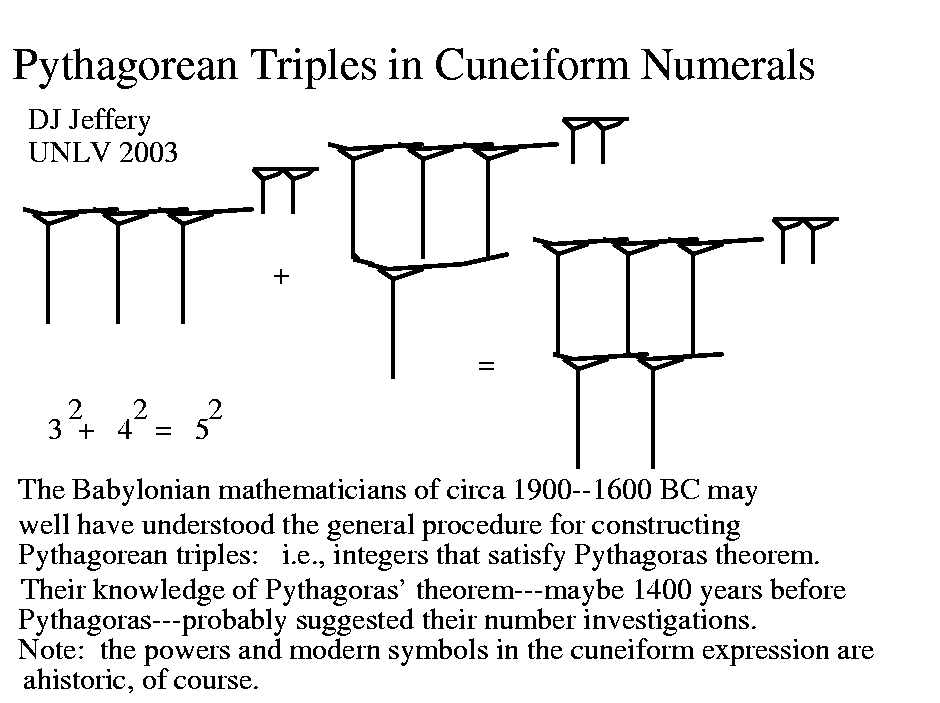
3. Image 3 Caption: Pythagorean triples and Pythagorean theorem.

   Babylonian mathematics went beyond simple arithmetic.

   For example, the Babylonian mathematicians knew the Pythagorean theorem (Ne-36) and how to solve quadratic equations (Ne-41) before circa 1600 BCE.

   

   The Image 3 illustrates Pythagorean triples: 3 integers that satisfy the Pythagorean theorem in modern form and in pseudo Babylonian math form. The Babylonian math form is "pseudo" because Babylonian mathematicians would NOT have used exponents, plus signs, and equal signs. They must have conveyed the same information, but probably in a very klutzy way.

   From many cuneiform clay tablets, it is clear that the Babylonian mathematicians at least after circa 19th century BCE knew the Pythagorean theorem---and more than maybe 1400 years before Pythagoras (c.570--c.495 BCE) too (Ne-35--36). However, the Babylonian mathematicians probably did NOT know a general proof nor probably think such a thing needed. Pythagoras is credited with having discovered Pythagorean theorem: this may be just a legend, he may have made an independent discovery, or he may introduced it to Greco-Roman world. There is NO evidence from Greco-Roman antiquity that he gave a proof (see Wikipedia: Pythagoras: In mathematics).

   As for Pythagorean triples themselves, Babylonian mathematicians did investigate them. A famous cuneiform clay tablet Plimpton 322 strongly suggests that they knew a general procedure for constructing Pythagorean triples (Ne-40). Plimpton 322 gives a demonstration of the procedure it seems: it does NOT give any directions. Plimpton 322 was first deciphered by Otto Neugebauer (1899--1990) and Abraham Sachs (1915--1983) in Mathematical Cuneiform Texts Amer. Oriental Series 29. American Oriental Society, New Haven, 1945 (see relevant p. 38). The above interpretation of Plimpton 322 has been disputed (see Wikipedia: Plimpton 322: Interpretations). Plimpton 322 is part of the G.A. Plimpton Collection of Columbia University.

   Of course, the Babylonian mathematicians did NOT know a lot of things. For example, they did NOT have the modern compact mathematical notation we have and they did NOT have the concept of using symbols to represent general numbers and unknowns. No one did until the 15th century and 16th century (see Wikipedia: History of mathematical notation; Wikipedia: History of mathematical notation: Symbolic stage; Wikipedia: Timeline of algebra).

Images:

1. Credit/Permission: © David Jeffery, 2003 / Own work.
   Image link: Itself.
   
2. Credit/Permission: © David Jeffery, 2003 / Own work.
   Image link: Itself.
   
3. Credit/Permission: © David Jeffery, 2003 / Own work.
   Image link: Itself.