- Continuous spectra
are of little use in
determining radial velocities
since it hard to impossible to know the
shape of the continuous spectra
in their emission reference frame.
- On the other hand,
line spectra
(either emission line spectra
or absorption line spectra)
are easily used.
The observed
line spectra
are Doppler shifted
from their emission reference frame,
but you can recognize the line pattern, and so
identify the
atomic lines
or molecular lines.
The known
rest frame
wavelengths
of the
atomic lines
or molecular lines
are, in fact,
their
emission reference frame
wavelengths---and so these are known too.
- Once you have an
emission reference frame
wavelength, one can
determine the radial velocity from
the relativistic Doppler effect
formula
for wavelength shift
λ_2 = λ_1 * sqrt[(1+v/c)/(1-v/c)] ,
where λ_2 is the observed line wavelength, λ_1 is the emitted line wavelength, the vacuum light speed c = 2.99792458*10**8 m/s (exact by definition) ≅ 3*10**8 m/s = 3*10**5 km/s ≅ 1 ft/ns, and v is the radial velocity to the astronomical object. Note v is positive/negative for recession/approach.
- The inverse function for
v/c is
v/c = [(λ_2/ λ_1)**2 - 1]/[(λ_2/ λ_1)**2 + 1] ,
- If |(λ_2/ λ_1)-1| << 1,
you can use
1st order Doppler effect formula
Δλ/λ = v/c ,
to determine v/c. This is done in the example calculation in the image.
- Note that extragalactic sources
outside of the
Local Group of Galaxies
have a
cosmological redshift
as well as a
Doppler shift
and the
cosmological redshift
grows with distance
and becomes dominant beyond ∼ 5 Mpc
(see
Wikimedia Commons:
File:Hubble constant.JPG).
Beyond ∼ 100 Mpc, the
Doppler shift
may become negligible depending on your
needed accuracy/precision.
Note the cosmological redshift is NOT a Doppler shift in the opinion of yours truly though they are related effects. They have different formulae, except to 1st order in small v/c. Some people call the cosmological redshift a Doppler shift, but yours truly thinks this is wrong.
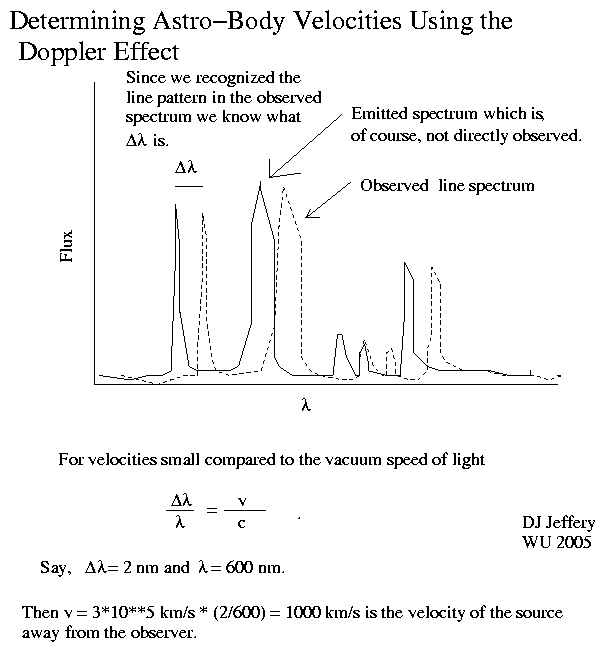
Caption: Determining the line-of-sight velocity or radial velocity to astronomical objects from the Doppler effect and line spectra.
Features:
Local file: local link: doppler_effect_line_spectra.html.
File: Waves file: doppler_effect_line_spectra.html.