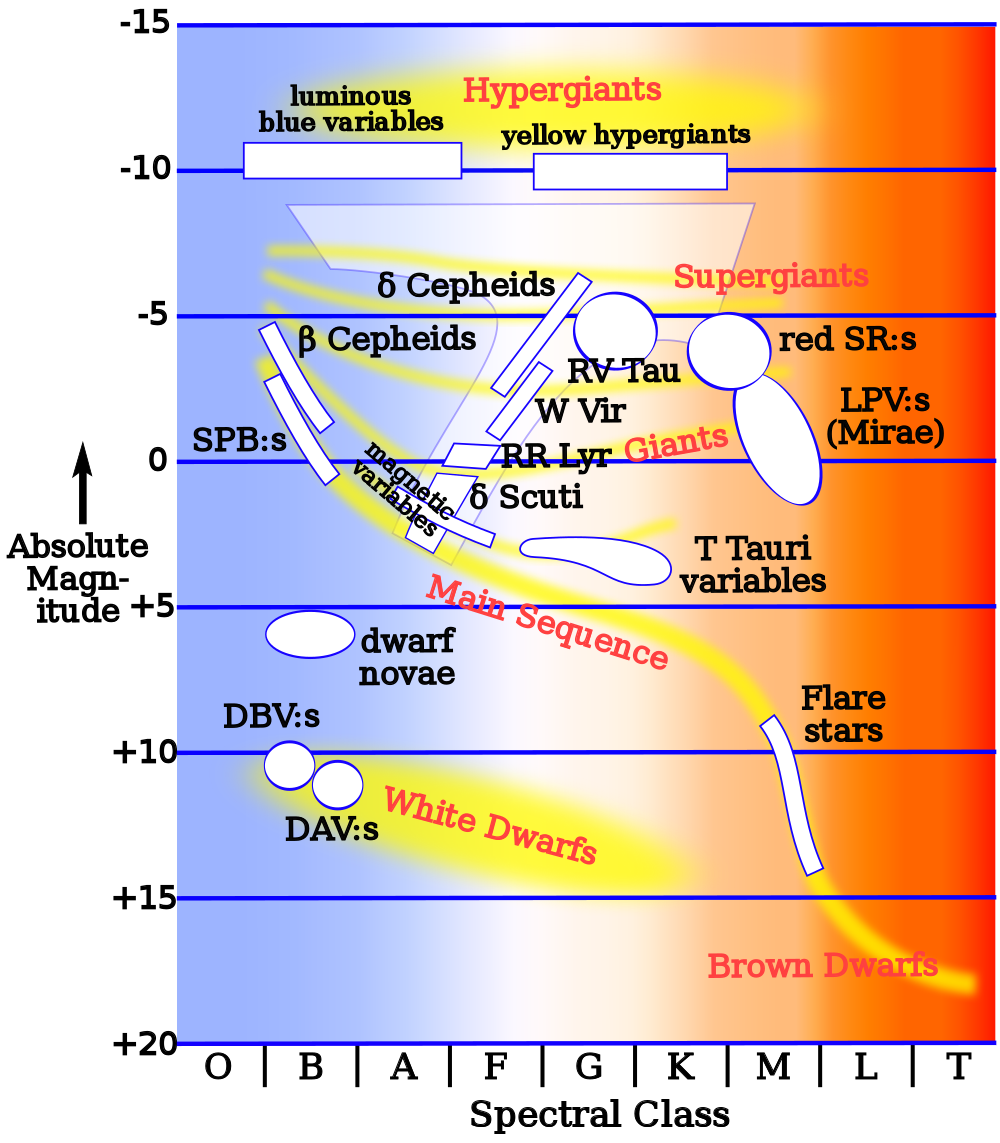
Image 1 Caption: A representative Hertzsprung-Russell (HR) diagram showing variable stars including Cepheids.

Features:

The key variation of variable stars is variation in luminosity: other quantities will vary too. All variable stars with large variations are post-main-sequence stars yours truly thinks.
There are several classes of Cepheids (Wikipedia: Cepheid variable: Classes), in fact, but we will NOT go into that complication very much here.
The key point is that Cepheids of each main Cepheid class have a definite period-luminosity relation: logarithmic mean luminosity is linear with the logarithmic period of the luminosity variation.
Image 2 Caption: A representative semi-log plot (alas NOT a log-log plot) of the period-luminosity relation for the 2 main Cepheid classes: the blue curve for classical Cepheids (which are relatively high metallicity Population I stars) and the green curve for Type II Cepheids (which are old, relatively low metallicity Population II stars).
The y-axis is absolute magnitude which is logarithmic luminosity in a obscure way where more negative is higher luminosity.
The x-axis is period in days (i.e., in units of the standard metric day = 24 h = 86400 s).
Actually, classical Cepheids and Type II Cepheids are quite different stars though they are both post-main-sequence stars (and so actually in brief phases of their lifetimes as nuclear burning stars) and have the same basic pulsation mechanism that causes their variability (see Wikipedia: Pulsation model; Kappa-mechanism).
"Classical Cepheids have stellar mass in the 4--20 M_☉ and have luminosity usually in the range ∼ 1,000 to 50,000 L_☉. They are bright giants or low luminosity supergiants in the spectral type range F6 -- K2" (somewhat edited from Wikipedia: Classical Cepheid variable: Properties). The lifetimes as nuclear burning stars of the classical Cepheid progenitors are <∼ 400 Myr (see Star file: star_lifetimes.html). Because of the relatively short lifetimes of their progenitors, classical Cepheids are likely to be very rare in elliptical galaxies which usually are or nearly are quenched galaxies.
Type II Cepheids are thought to have stellar mass ⪅ 1 M_☉ (see Wikipedia: Type II Cepheid: Properties), and so their progenitors have lifetimes as nuclear burning stars of ⪆ 10 Gyr (see Star file: star_lifetimes.html). In fact, Type II Cepheids are thought to be typically ∼ 10 Gyr old (see Wikipedia: Cepheid variable: Type II Cepheids). Because of their age Type II Cepheids can be found in globular clusters which have ages in the range 12??? --- 12.7 Gyr (see Wikipedia: Globular clusters: Consequences) and in quenched galaxies like most elliptical galaxies.
The period-luminosity relation of Cepheids was discovered by Henrietta Swan Leavitt (1868--1921) in 1908 at Harvard College Observatory while working as "computer". Nowadays we use electronic computers, in those days we used women.
From period-luminosity relation, a measurement of the period gives the mean luminosity from which distance can be determined using inverse-square law formula (3) below:
1. L = F*(4πr**2) gives luminosity L for measured radiant flux F and distance r.
2. F = L/(4πr**2) gives radiant flux F for known luminosity L and distance r.
3. r = sqrt[L/(4πF)] gives distance r for known luminosity L and measured radiant flux F.
The period-luminosity relations for the 2 main Cepheid classes have to be empirically calibrated from Cepheids at known distances so that their luminosities can be determined from inverse-square law formula (1) given above.
Uncertainties in the empirical calibrations, other measurement uncertainties, and intrinsic scatter in Cepheid behavior leads to uncertainties in Cepheid distance determinations---which are still a significant problem circa 2020s---but, of course, the absolute size of the uncertainties are much smaller now than in the days of Henrietta Swan Leavitt (1868--1921), but our requirements for accuracy/precision are much higher.
Despite uncertainties and other complications, Cepheids are a key cosmic distance indicator for distances from 100s of parsecs to ∼ 30 megaparsecs currently because they are very luminous: their luminosities are in the range ∼ 3*10**2 -- 5*10**4 L_☉. They be can observed in nearby and not-quite-nearby galaxies.
The range of Cepheids as a cosmic distance indicator has been considerably extended by the Hubble Space Telescope (HST, 1990--2040?, d = 2.4 m, Cassegrain reflector) and James Webb Space Telescope (JWST, 2021--2041?, diameter = 6.5 m, 18 segment mirrors of gold-plated beryllium, Cassegrain reflector) Hubble Space Telescope (HST) because of their great resolution: i.e., they can resolve Cepheids to large distances. Observing Cepheids at greater distances should become possible in the future.
Cepheids are a key rung in the cosmic distance ladder and have been used in modern physical cosmology since its early days in the early 20th century.
Speaking of the early days, Edwin Hubble (1889--1953) assumed that there was only one kind of Cepheid, NOT two. So in fact in he used the calibration for Type II Cepheids when observing classical Cepheids in other galaxies (Wikipedia: Hubble's law: Earlier measurement and discussion approaches). Now to relate the luminosity of the two kinds, L_classical ≅ 4L_Type_II. Thus, according to formula (3) cited above
```
          r = sqrt[L/(4πF)]  , 
```
correcting from L_Type_II to L_classical caused, all early cosmic distance determinations to increase by roughly a factor of 2.
Making the correction to classical Cepheids luminosities reduced in the Hubble constant from ∼500 (km/s)/Mpc to ∼250 (km/s)/Mpc in 1952 (Wikipedia: Hubble's law: Earlier measurement and discussion approaches). Other corrections reduced the Hubble constant to its current fiducial value of ∼ 70 (km/s)/Mpc (see Wikipedia: Hubble's law: Determining the Hubble constant). The major modern uncertainty with the Hubble constant is the Hubble tension (direct value ≅ 73(1) (km/s)/Mpc; Λ-CDM fit value 67.5(10) (km/s)/Mpc). The Hubble tension may lead to a revision of Λ-CDM model or its replacement as the standard model of cosmology (SMC, Λ-CDM model).
If this is the first time this insert is used in a file, you will see for general reference, the main-sequence rule below (local link / general link: star_main_sequence_rule.html).

Images:

Credit/Permission: © User:Rursus, 2008 / Creative Commons CC BY-SA 3.0.
Image link: Wikimedia Commons: File:HR-vartype.svg.
Credit/Permission: User:Vedran_V, 2014 / Public domain.
Image link: Wikimedia Commons: File:Period-Luminosity Relation for Cepheids.png.

Local file: local link: star_hr_cepheids.html.
File: Star file: star_hr_cepheids.html.