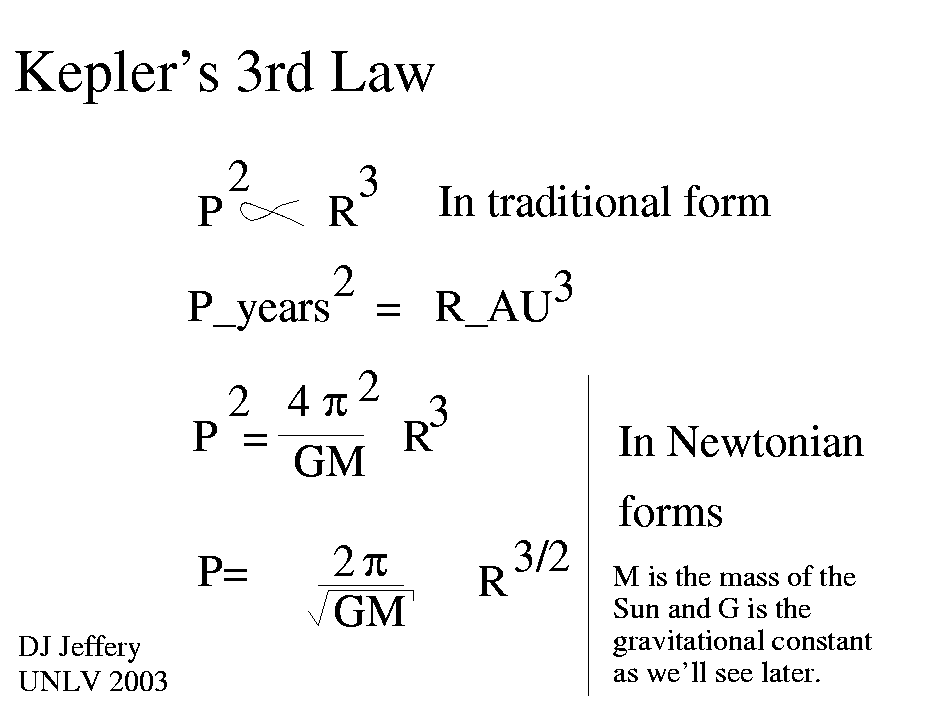
Caption: Kepler's 3rd law and the Newtonian Kepler's 3rd law formula illustrated.

Kepler's 3rd law applies to secondary bodies (or orbiting bodies) orbiting a primary body. The law is exact in the limit that each secondary body and the primary body is an exact gravitationally-bound gavitational two-body system.

The law assumes that the secondary body mass is negligible compared to the primary body mass, unless the statement of the law explicitly says its NOT negligible.

Kepler's 3rd law can written any number of ways:

1. The orbital period p squared is proportional to the mean orbital radius r cubed. The mean orbital radius (AKA the semi-major axis) r is measured to the center of the primary body.

2. p**2 ∝ r**3.

3. p_yr**2 = r_AU**3, where p_yr is orbital period in sidereal years and r_AU is mean orbital radius in astronomical units (AU). This formula applies only to Solar System planets since the general formulae depend on the primary body mass (see Items 5 and 6 below).

4. p_yr = r_AU**(3/2) which is the same as the last formula, except both sides have been square rooted.

5. Newtonian Kepler's 3rd law formula p = [(2π)/sqrt(GM)]*r**(3/2), where M is the mass of the primary body in a gravitationally-bound gavitational two-body system, the mass of the secondary body is negligible compared to M, and G is gravitational constant G=6.67384*10**(-11) (MKS units).

6. Newtonian Kepler's 3rd law formula p = [(2π)/sqrt(G*(M+m))]*r**(3/2) (general case) for the case where the mass m of the secondary body in the gravitationally-bound gravitational two-body system is NOT negligible compared to M. In this case, r is the relative mean orbital radius (see Wikipedia: Standard gravitational parameter: General case).