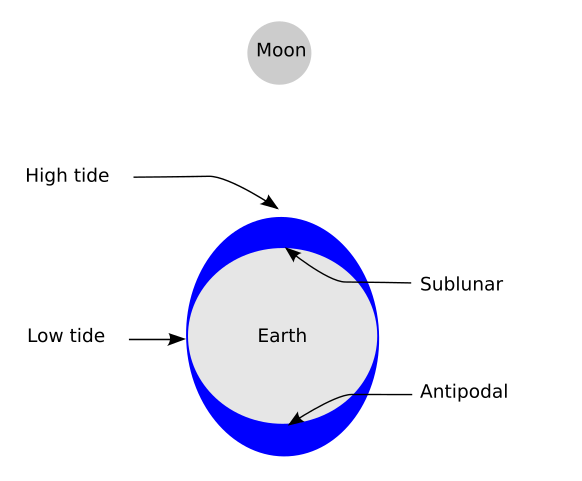
General Caption: In this figure, we analysis the tides on Earth in the ideal limit: the ideal tide. The ideal tide gives the main behavior---all the other behaviors are perturbations---but very noticeable ones on the human scale when you are NOT in the open ocean.

The analysis is well beyond the scope of an introductory astronomy course and yours truly wishes someone had explained it to yours truly in 1977. Read it as poetry.

Features:

Image 1 Caption: The ideal tide caused by the Moon alone. There is the rigid spherically-symmetric solid-phase Earth and and incompressible fluid world ocean. The world ocean's symmetrical tidal bulges are caused by the Moon's tidal force. There is axial symmetry about the Earth-Moon line. The sublunar point and its antipodal point are on this line. The world ocean's geometric shape is NOT, in fact, a prolate spheriod (AKA ellipsoid of revolution) though that geometric shape does turn up in another ideal case of tidal force effects (see Wikipedia: Tidal force: Effects).
Image 1 vastly exaggerates the size of the tidal bulges. As we show below, the ideal tidal range is 1.07145 m and the observed open ocean tidal range is typically ∼ 0.6 m.
First, we need the appropriate physics:
1. Any astro-body that interacts with the rest of the universe only through gravity has an associated inertial frame (i.e., a free-fall frame) that moves with its center of mass (CM) which it is usually convenient to define as the origin of inertial frame coordinates.
2. All the internal motions of the astro-body can be analyzed in this CM inertial frame using Newtonian physics as long as the astro-body is in the classical limit:
  1. Relative velocities much less than the vacuum light speed c = 2.99792458*10**5 km/s.
  2. Gravitational field much less than near black holes.
  3. Total mass-energy of the astro-body much less than that of the observable universe.
  4. Size scale in the analysis much larger than the size scale of atoms and molecules.
  The classical limit sounds very restrictive, but pretty much everything from cosmic dust to the large scale structure can be analyzed as in the classical limit in good to excellent approximation. Black holes CANNOT of course.

We can prove the 2 statements made just above about the CM inertial frame from Newtonian physics plus an extra confirmation from modern general relativistic cosmology.

Proof:

First, let position r, velocity acceleration a, force F, and gravitational field g be vectors even though we do NOT indicate that in the notation. Mass m is a scalar, of course. All of these dynamical variables have extra adornments as needed.
Let an astro-body be divided into particles i (i.e., physical objects of negligible size and internal structure) of mass m_i, and acceleration a_i, acted on by force F_i, and gravitational field g_i.

Newton's 2nd law of motion (AKA F=ma) applied to particle i gives

  m_i*a_i = F_i = F_i' + m_i*g_i  where F_i is the net force on particle i,
                                        F_i' is the net internal force on particle i
                                          (including internal gravitational fields),
                                        and g_i is the external gravitational field at particle i
                                          due to the whole rest of the universe. 
                                        In fact, in most cases, g_i can be calculated by integrating
                                        up the gravitational fields
                                        due to relatively local sources of gravity
                                        using just Newtonian physics.
                                        More remote sources can be assumed to cancel
                                        due to the uniform density of the universe on average:
                                        a source far off in one direction will be canceled
                                        by one far off in the opposite direction.
                                        This procedure is invalid for the observable universe 
                                        as whole for which general relativistic cosmology is needed.
                                        General relativistic cosmology gives us the comoving frames
                                        that expand with the observable universe
                                        and it confirms the
                                        astrophysical 2nd law formula (A2LF)
                                        we derive here given the assumptions we made above 
                                        for large astro-bodies (e.g., galaxies and larger)
                                        that are just moving with a comoving frame
                                        (i.e., participating in the mean expansion of the universe). 
                                        This is the extra confirmation alluded to above. 
                                        Smaller astro-bodies are part of larger ones,
                                        and so the confirmation propagates down to all
                                        smaller scales from the comoving frame scale.

  F_i' = sum_j(F_ji)              where F_ji is the force of particle j on particle i.

  We sum on i to get
 
  sum_i(m_i*a_i) = (1/2)sum_(ij)F_ij + sum_i(m_i*g_i) = sum_i(m_i*g_i)

    where the (1/2) is to correct for double counting and

    (1/2)sum_(ij)F_ij = 0 by pairwise cancellation of forces by Newton's 3rd law. 

  Now the CM is defined by r = sum_(m_i*r_i)/m, where m = sum_i(m_i), and thus we get

    v = sum_(m_i*v_i)/m and a = sum_(m_i*a_i)/m.

  Note that origin and absolute size scale for r_i, v_i, and a_i are left
    unspecified since we do not need those specifications, but they could be specified
    relative to a local comoving frame if needed.

  Now, we define g_ave = sum_i(m_i*g_i)/m = a to be mass-weighted average external gravitational field.

  We can now write Newton's 2nd law for particle i as

  m_i*a_i = sum_j(F_ji) + m_i*g_i

  m_i*(a_i-a) = sum_j(F_ji) + m_i*(g_i - a)

  m_i*(a_i-a) = sum_j(F_ji) + m_i*(g_i - g_ave)

  m_i*a_i' = sum_j(F_ji) + m_i*[ (g_i-g) - sum_j[(m_j)*(g_j-g)/m] ] ,

  where

  m_i*a_i' = sum_j(F_ji) + m_i*gt(i) is our final 

    astrophysical 2nd law formula (A2LF),

    where a_i' is acceleration relative to the CM, 

    g is the external gravitational field at the CM,

    gt(i) = [ (g_i-g) - sum_j[(m_j)*(g_j-g)/m] ]  
 
    is the effective tidal field 
       (i.e., force per unit mass) on particle i,
 
    and the A2LF
            makes no use of absolute position, velocity, and acceleration.
            So those can all be calculated relative to the CM.

    A tidal force is just the difference in gravity between two points.

The existence of the astrophysical 2nd law formula (A2LF) completes the proof. If a form of the Newton's 2nd law of motion (AKA F=ma) holds for the CM defined reference frame, then it is an inertial frame in which Newtonian physics holds.

Note the 2nd term in the effective tidal field is annoying. However, it is probably often small or negligible due to cancellation by significant symmetry of the astro-body and of the tidal field. In fact, for the ideal tide, it is zero by symmetry as we show below.

Note that the tidal forces are the only external gravitational effect on the internal motions of the astro-body.
The following par is hogwash---correct it.
This is good because the g_i CANNOT be calculated correctly by Newtonian physics as noted above. However, the tidal forces can be. Asymptotically with increasing distance r (i.e., distance from the CM recall) from an external gravity source, the tidal force declines in size as 1/r**3 (which we actually show below for the special case of the ideal tide). Now given the 1/r**3 decline, one can integrate up the tidal forces extending to infinity in a universe of uniform density on average and get a finite value. (We do NOT know if the universe is infinite, but it does seem to have uniform density on average. See Wikipedia: Cosmological principle.)
In fact, usually cosmologically remote contributions are negligible (which is good since we CANNOT calculate them correctly by Newtonian physics) and for many (but NOT all) systems, all one needs is calculate the tidal forces from a few nearby sources of gravity. In fact, for many important systems, the tidal forces are completely negligible. If the astro-body is small enough, the external gravitational field is approximately uniform and the tidal forces approximately zero. This is true of spacecraft in orbit.
The A2LF we have derived can be applied to many astro-bodies: artificial satellites, moon systems, planetary systems, nebulae, star clusters, galaxies, galaxy clusters, and galaxy superclusters. So there is a whole hierarchy of astro-bodies within astro-bodies it can be applied too. Of course, there might complicated astro-bodies for which another approach even when Newtonian physics applies when something other than the A2LF may be more expedient. The A2LF does NOT apply to black holes or the universe as whole. For those you need general relativity.
Actually, if you want very accuracy/precision, you need general relativity even for systems where the A2LF gives very good results. The tiny perihelion shift of Mercury required general relativistic treatment to be accounted for. The that accounting was the first evidence for general relativity (see Wikipedia: Tests of general relativity: Perihelion shift of Mercury).

Some more general explication is needed:
1. Note that inertial frames (i.e., free-fall frames) do NOT rotate with respect the observable universe: i.e., with respect the bulk mass-energy observable universe, and therefore with respect to each other. The last statement is, of course, as far as we can tell. However, even it is NOT exactly true, it may be an exact emergent theory in the limit of the observable universe.
  EXCEPT that very strong gravitational fields (like those very near black holes) may cause the rotation of free-fall frames relative to the observable universe, but this is a tricky point for which yours truly cannot find a clear explication. The best so far (and it does NOT say much) is Wikipedia: Inertial frame of reference: General relativity.
  The upshot is that there is an absolute rotation (i.e., one with respect to the observable universe) even though there is NO absolute space in the sense used by Isaac Newton (1643--1727).
2. Isaac Newton (1643--1727) did NOT have our modern view inertial frames: i.e., that are free-fall frames. He believed that the fixed stars were on average at rest in his absolute space which was the fundamental inertial frame. Note that for Newton the fixed stars were just the stars very nearby in Milky Way which he did NOT know was a galaxy in our sense. All reference frames NOT accelerated relative to absolute space were also inertial frames and all reference frames accelerated with respect to absolute space were non-inertial frames (see Wikipedia: Inertial frame of reference: Newton's inertial frame of reference).
  Nowadays, we do know inertial frames (i.e., free-fall frames) attached to centers of mass (CMs) do accelerate with respect to each other. This follows from the derivation and form of the A2LF above.
  But local inertial frames (i.e., those at the same place) do NOT accelerate with respect to each other: they only differ in velocity. Reference frames that accelerate relative to local inertial frames are our modern non-inertial frames.
  However, despite original Newtonian physics view of inertial frames and non-inertial frames that was apparently held up until the advent of general relativity in 1915 people were able before 1915 to use Newtonian physics to calculate to high accuracy/precision the behavior Solar System, Earth-Moon system, moon systems and the tides (see Wikipedia: Tide: History of tidal theory; Theory of tides: History).
  Yours truly has to think those old old-time celestial mechanics practitioners were somehow using our derived A2LF implicitly assuming it was right or that non-inertial-frame effects were negligible. But this is a fine point of the history of celestial mechanics.
3. In our modern view, there is no ONE fundamental inertial frame like Newton's absolute space. The modern view is that the expanding universe (i.e., the expanding observable universe) provides a continuum of fundamental inertial frames (i.e., free-fall frames) called comoving frames. But all other free-fall frames are inertial frames too and there is a whole hierarchy of them because there is a whole hierarchy of orbits and the centers of mass of the orbiting astro-bodies that are in free fall. Recall, we discussed this above.
4. How does one deal with non-inertial frames? inertial forces
The Earth's center of mass CM_⊕ is in free fall and therefore defines an (exact) inertial frame (i.e., a free-fall frame). This follows from our modern understanding inertial frames as told to us by general relativity (GR) via the strong equivalence principle.
Why is the center of mass of an astro-body in free fall?
Well, if the only external force on the astro-body is gravity (i.e., the only significant one), then Newtonian physics (which is good enough for our understanding here) dictates that its center of mass is in free fall. The internal forces on the astro-body (e.g., self-gravity, pressure force, etc.) cancel out pairwise by Newton's 3rd law of motion as far as the motion of the center of mass is concerned. Internal forces AND external forces too can cause motions relative to the center of mass, of course.
A key point about free-fall frames is that the gravitational force at the and the inertial force (which is an effect of acceleration relative to all local inertial frames) cancel exactly it seems. Albert Einstein (1879--1955) took the exact cancelation as exactly true and based the Einstein equivalence principle (which can be strengthened to the strong equivalence principle) on this. This principle states no local experiment can distinguish between the gravitational force and the inertial force. So somehow they are fundamentally the same thing---they do the same things. So yours truly finds it philosophically congenial to use inertial forces whenever it is convenient---if you do all the analysis right, you always get the right answer.
Now one can extend the use of the CM_⊕ inertial frame to include the whole Earth for the analysis of the Earth's motions relative to the CM_⊕ and determination of its shape.
To do so one must regard deviations in the external gravitational field (see definition below) from the external gravitational field at the CM_⊕ as forces in their own right. They are called tidal forces. Clearly tidal forces are combinations of gravitational forces and inertial forces, but as follows from the discussion above that in itself causes no errors in analysis.
Note the tidal force at the CM_⊕ is by definition zero
Note the extension of the CM_⊕ inertial frame to the whole Earth for the analysis is valid, but extending it further it becomes increasingly less convenient for analysis to the point that it becomes horrifically inconvenient and as relativistic effects become important increasingly wrong. The perspective of the expanding universe with its continuum of comoving frames (i.e., the basic of inertial frames) is the best perspective for the analysis of the observable universe so far as we know.
The external gravitational field is provided by whole universe (so far as we know) external to the Earth. However, the most significant deviations are provided by the gravity of the Moon and secondarily the Sun. Other Solar System astro-bodies provide very minor deviations though they do have noticeable effects. Astro-bodies from beyond the Solar System give completely negligible deviations.
The Earth's center of mass CM_⊕ is in free fall and therefore defines an (exact) inertial frame (i.e., a free-fall frame). This follows from our modern understanding inertial frames as told to us by general relativity (GR) via the strong equivalence principle.
Image 2 Caption:
The tides can be analyzed to good 1st order accuracy/precision by an expansion of the external gravitational field (i.e., gravitational force per unit mass) of an external spherically symmetric source of gravity (i.e., the Moon or the Sun) about the CM_⊕:
1. Let r be the radius coordinate from the external source center.
2. Let r_cm be the radius coordinate from the external source center to the CM_⊕. This radius defines the symmetry axis of the system.
3. Let x be the coordinate of the symmetry axis increasing away from the external source center with origin at the CM_⊕.
4. Let y be the coordinate of axis perpendicular to the symmetry axis with origin at the CM_⊕.
5. The x and y coordinate is in a particular plane through the symmetry axis, but by symmetry all other such planes will give the same behavior.
6. The x and y components of the external gravitational field are:
```
       
  g_x = (-GM_ext/r**2)*cos(γ) = [-GM_ext/((r_cm+x)**2+y**2)]*[(r_cm+x)/sqrt((r_cm+x)**2+y**2)]

  g_y = (-GM_ext/r**2)*sin(γ) = [-GM_ext/((r_cm+x)**2+y**2)]*[y/sqrt((r_cm+x)**2+y**2)] 
```
7. We now make 1st order expansions in small (x/r_cm) and (y/r_cm). This is highly accurate/precise since (x and y) ≤≅ R_eq_⊕ << r_cm. This means that the 2nd-order corrections terms (x/r_cm)**2 << 1 and (y/r_cm) << 1 and they (and higher order corrrection terms) can be neglected. To be explicit, note that R_⊕/r_cm = 0.0165774 = 1/60.3229 ≅ 1/60 for the Moon as the external source and R_⊕/r_cm = 4.2635169*10**(-5) ≅ 1/(2.5*10**6) for the Sun as the external source. The large number of significant figures follows from using for the sake of definiteness/consistency high accuracy/precision input values: Earth equatorial radius R_eq_⊕ = 6378.1370 km, Moon mean orbital radius R_orbital_Mo = 384,748 km = 60.3229 R_eq_⊕ ≅ 60 R_eq_⊕, and Earth mean orbital radius R_orbital_⊕ = 149,598,023 km. We the same accuracy/precision input values in following calculations for the same reasons.
  The relevant expansions and resulting relevant formulae are:
```
  r**2   = (r_cm+x)**2+y**2) = r_cm**2*[1+2*(x/r_cm)+(x/r_cm)**2+(y/r_cm)**2] ≅ r_cm**2*[1+2*(x/r_cm)]

  1/r**2 ≅ (1/r_cm)**2*[1-2*(x/r_cm)] 
  r      ≅  r_cm*[1+(x/r_cm)] = r_cm + x
  cos(γ) ≅ 1
  sin(γ) ≅ y/r_cm

  g_x_1st = (-GM_ext/r_cm**2)*[1-2*(x/r_cm)]
  g_y_1st = (-GM_ext/r_cm**2)*(y/r_cm)

  gt_x_1st = (2GM_ext/r_cm**3)*R_eq_⊕*(x/R_eq_⊕)   1st order x-coordinate tidal force
  gt_y_1st = (2GM_ext/r_cm**3)*R_eq_⊕*(-1/2)*(y/R_eq_⊕)   1st order y-coordinate tidal force

  gt_strength = (2GM_ext/r_cm**3)*R_eq_⊕  fiducial tidal force strength
  gt_strength_Moon = 1.09753*10**(-6) N/kg = 1.11992*10**(-7)*g   fiducial tidal force strength for the Moon.
  gt_strength_☉ = 0.505695*10**(-6) N/kg = 0.516015*10**(-7)*g  fiducial tidal force strength for the Sun.  
```
  where g is Earth's surface gravitational field g (fiducial value 9.8 N/kg). We can see that gt_strength_Moon and gt_strength_☉ are very small compared to g which means local variations on the surface of the Earth of the external gravitational field have very small and usually negligible effect. However, over the extend of the Earth, they give the tides.
Image 3 Caption:
We will now calculate the ideal oceanic tide. We assume that the solid Earth is a rigid sphere with Earth equatorial radius R_eq_⊕ = 6378.1370 km. The sphere has a layer of water (i.e., an ideal world ocean) with the water assumed to act as an ideal incompressible fluid. We assume that world ocean has reached hydrostatic equilibrium. This is an approximation clearly since the Earth and external source are moving in the CM_⊕ inertial frame. The approximations implied by the above assumptions are large, but nevertheless the calculated tidal range is of order of the actual open ocean tidal range.
We need a differential equation for pressure along the solid Earth from the point (x=R_e_⊕,y=0) to the point (x=0,y=R_e_⊕,y=0). The appropriate differential formula for volume element of volume dV and cross-sectional area dA is
```
  (p_b - p_a)*dA = ρ*g_θ*dV   where ρ is water density assumed constant,

                                    θ is the angle subtended at the CM_⊕ by the arc
                                      length along the solid Earth measured from the positive
                                      x axis counterclockwise toward the positive y axis,


                                         b and a are points on the solid Earth, θ_b > θ_a.

   g_θ =  -ρ*gt_x_1st*sin(θ) + ρ*g_y_1st*cos(θ) is the tidal field along the
                                                              solid Earth increasing θ as the 
                                                              positive direction.  Thus g_θ
                                                              points in the negative direction.  
```
The differential equation follows and solution follows:
```
                                          
 (dp/dθ) = -R_eq_⊕*ρ*gt_strength*[cos(θ)*sin(θ) + (1/2)*sin(θ)*cos(θ)]

  p(θ) = p_x_axis - (3/2)*R_eq_⊕*ρ*gt_strength*sin(θ)**2 
```
To convert to sea level h, we assume the only significant gravitational field which points radially downward and that air pressure p_air is constant. The formula for pressure with depth is
```
  p = p_air + ρ*g*h  
```
(see Wikipedia: Hydrostatics: Pressure in fluids at rest). Since pressure in hydrostatic equilibrium is isotropic (i.e., the same in all directions), combining the last two equations we obtain the following expressions
```
  h(θ) = h_x_axis - (3/2)*R_eq_⊕(gt_strength/g)*sin(θ)**2

  h_x_axis - h_y_axis = (3/2)*R_eq_⊕*(gt_strength/g)   tidal range in general

                      = 1.07145 m for the Moon alone
                      = 0.493682 m for the Sun alone.  
```
The values for the ideal tidal range we have just calculated agree to better than 2 significant figures with those cited by Wikipedia: Tide: Amplitude and cycle time which were doubtless calculated in a similar way, but probably with slightly different input numbers.
The observed open ocean tidal range is typically ∼ 0.6 m. Given the vast simplifying assumptions made for our ideal tidal ranges

Credit/Permission: User:Krishnavedala, 2014 / CC BY-SA 3.0.
Image link: Wikimedia Commons.

local link: tide_ideal.html

Mechanics file

tide_ideal.html