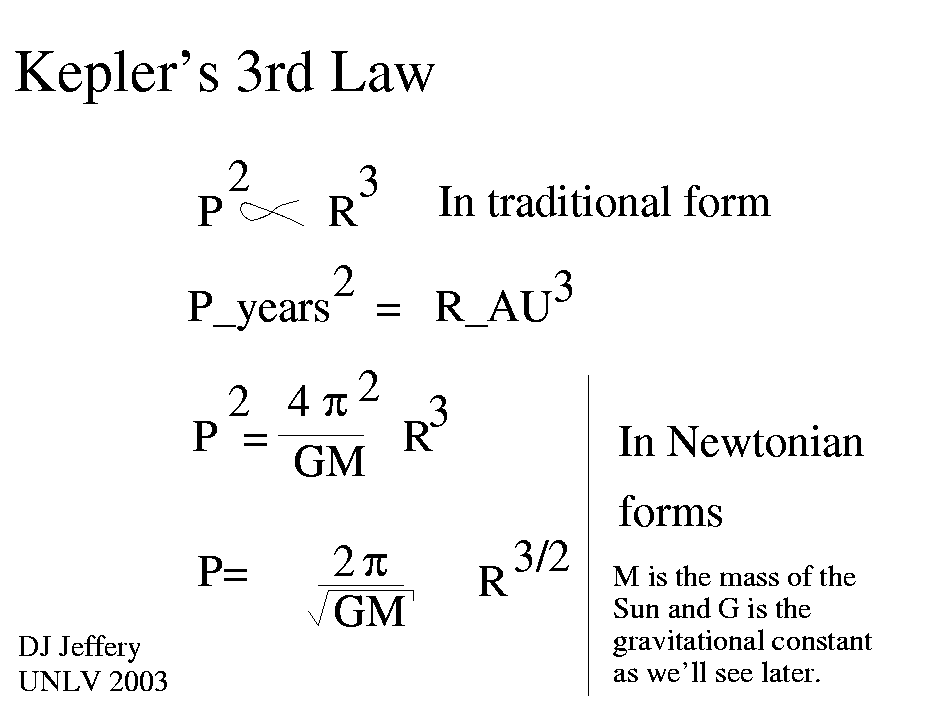
Caption: Kepler's 3rd law illustrated.

Features:

1. In words, Kepler's 3rd law states that the square of an orbital period of a planet is proportional to the cube of the mean orbital radius (AKA semi-major axis) of its elliptical orbits around the Sun. In natural units, the formula is

     P_years**2 = R_AU**3  , 

   where AU stands for astronomical unit (AU) ≡ 1.49597870700*10**11 m ≅ 1.496*10**11 m. Of course, for the Earth, we have 1 = 1 since the natural units are natural for us Earthlings.

2. Kepler's 3rd law was remarkable in showing there was a connection between the specific geometry of orbits (i.e., ellipses) and kinematics (i.e., which is concerned with the description of motion).

3. Isaac Newton (1643--1727) in his Principia (1687) derived Kepler's 3rd law from Newtonian physics (i.e., Newton's 3 laws of motion, Newton's law of universal gravitation, etc.) thereby connecting kinematics and dynamics (which is concerned with the causes of motion). The fact that gravity is an inverse-square law is a key factor in the derivation.

4. Note that Kepler's 3rd law only holds exactly for gravitationally bound two-body systems. However, for planetary systems and satellite systems (AKA planet-moon systems), it is usually the case that the host star and each planet in the first case and the host planet and each moon in the second case approximate a gravitationally bound two-body system to high accuracy/precision.

5. The Newtonian physics formula for Kepler's 3rd law is

     P = [(2π)/sqrt[G(M+m)]]*R**(3/2) ≅ [(2π)/sqrt[GM]]*R**(3/2) for m << M  , 

   gravitational constant G = 6.67430(15)*10**(-11) (MKS units), M is the mass of the larger spherically symmetric astro-body, and m is the smaller spherically symmetric astro-body. In natural units, the second version of the above formula becomes

     P_years**2 = [1/sqrt(M/M_☉)]R_AU**3  , 

   where the solar mass M_☉ = 1.98855*10**30 kg.

6. Johannes Kepler (1571--1630) published Kepler's 1st law and Kepler's 2nd law in Astronomia nova (New Astronomy) (1609). Kepler's 3rd law was discovered later and published in Epitome Astronomiae Copernicanae (Epitome of Copernican Astronomy) (1618--1621).