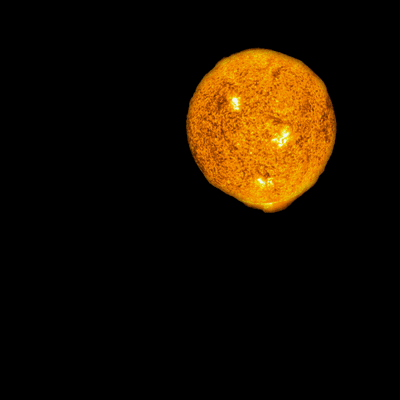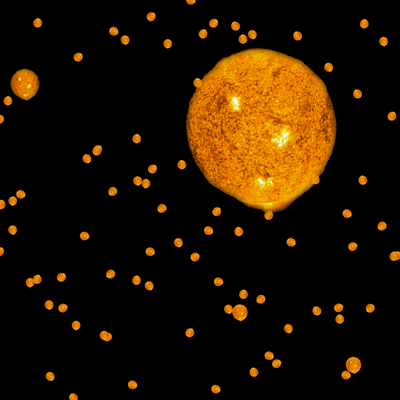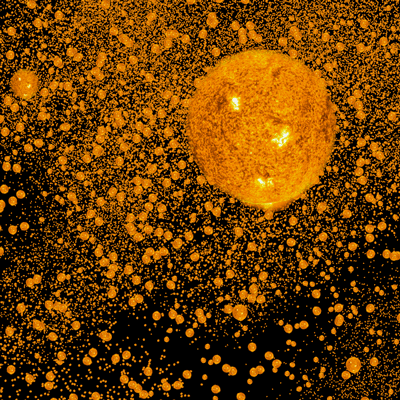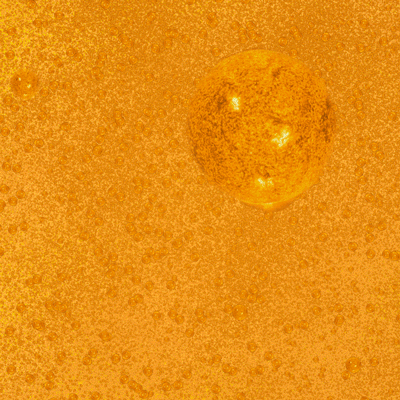
Caption: An animation illustrating Olbers' paradox (AKA dark night sky paradox) by filling the sky with stars and making the sky as bright as a star everywhere you look.

Features:

1. Olbers' paradox: If the universe is assumed (1) infinite, (2) eternal, (3) static, and (4) homogeneous, then in every direction you look in space you should see a star (more exactly a piece of stellar photosphere) and the sky should be as bright as a stellar photosphere---as the animation illustrates.

   Since we do NOT observe this super bright sky, one or more of the 4 assumptions is wrong.

2. The assumption that is certainly wrong is the static assumption since we observe the expansion of the universe meaning actually an expansion of the observable universe.

3. Actually, in the current cosmological paradigm Big Bang cosmology (which likely to be true as far as it goes), the observable universe began in a hot dense phase (i.e., Big Bang era) at lookback time the age of the observable universe = 13.797(23) Gyr (Planck 2018) (see Planck 2018: Age of the observable universe = 13.797(23) Gyr) and has been expanding since then. The observable universe is a sphere centered on us, the observers of the observable universe.

   Big Bang cosmology gives two causes for the blackness of space:

   1. Photons from beyond the particle horizon (i.e., the comoving radius of the observable universe = 14.25 Gpc = 46.48 Gly current value) have NOT had time to reach us since the Big Bang era. This is the main cause.

   2. The second cause is the expansion of the universe reducing the density of photons and, via the cosmological redshift, causing photons to lose energy (i.e., photon energy E = hf = hc/λ = 1.2398419739(75) eV-μ/λ_μ) as they propagate across intergalactic space.

   Both causes make the sky dimmer than otherwise.

4. Another perspective on Olbers' paradox is that the observable universe is NOT in thermodynamic equilibrium because of its finite age and the expansion of the universe.

   Counterfactually if it were in thermodynamic equilibrium, all astro-bodies would be at one temperature and the radiation field in space would be a blackbody radiation field at that one temperature.

5. In fact, the stars are trying to heat up the radiation field in space by emitting photons, but they are opposed by the expansion of the universe as described above.

   The heating up is a manifestation of the 2nd law of thermodynamics: photons are spontaneously (via random processes) flowing from the photon-dense environment inside stars to the photon-undense environment in space.

   If the Λ-CDM model is correct to infinite cosmic time (which is a wild extrapolation), the stars will NEVER succeed in heating up the radiation field in space and, in fact, thermodynamic equilibrium will be achieved at absolute zero asymptotically as cosmic time goes to infinity. This grand finale is the heat death of the universe.

6. The history of Olbers' paradox is rather intricate and goes back before Heinrich Wilhelm Matthias Olbers' (1758--1840) statements of Olbers' paradox in 1823 and 1826 (see Wikipedia: Heinrich Wilhelm Matthias Olbers: Life and career). In fact, the first modern statement of Olbers' paradox was published by Edmond Halley (1656--1742) in 1720 and reported in 1721 at a meeting of the Royal Society with none other than Isaac Newton (1643--1727) presiding as President of the Royal Society (1703--1727). Actually, Halley said that he heard of the Olbers' paradox from someone unnamed who may have been David Gregory (1659--1708) (see No-377,440). Lord Kelvin (1824--1907) in 1901 suggested a finite age for the universe as a possible resolution of Olbers' paradox since light from distant stars did NOT have time to reach us. This resolution is part of the modern one as discussed above. Remarkably, none other than Edgar Allan Poe (1809--1849) suggested the same resolution in 1848 (see Wikipedia: Olbers' paradox: History).