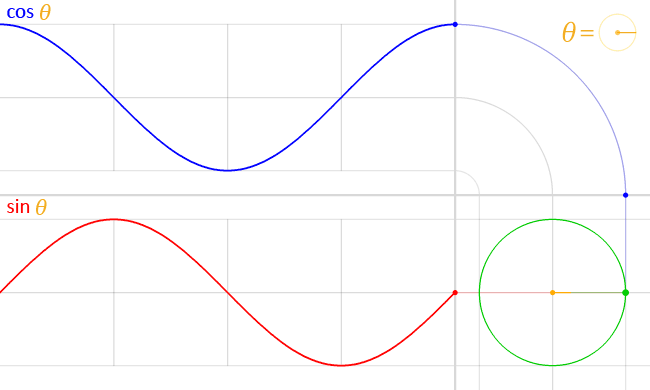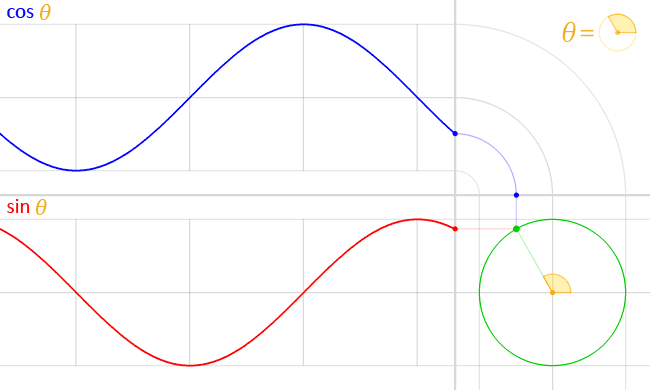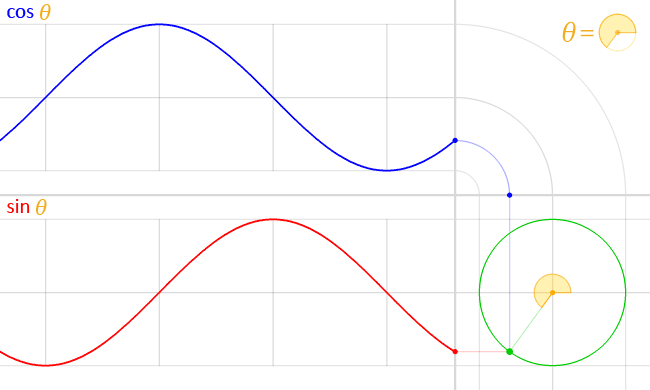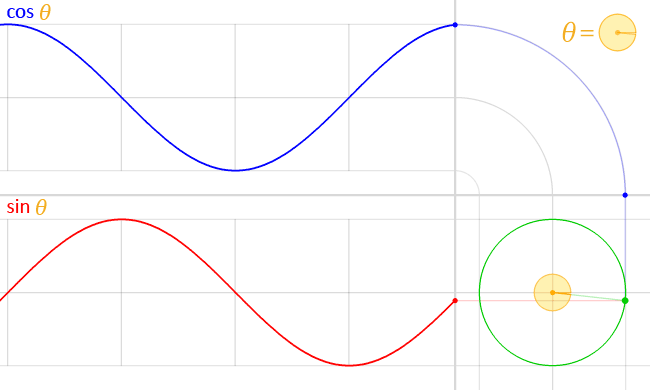
Caption: An animation illustrating how uniform circular motion relates to sinusoidal motion.

The horizontal/vertical projection of the uniform circular motion becomes the cosine function/sine function.

By inspection or symmetry if you prefer, it is clear that the two trigonometric functions are identical except that the cosine function has a phase shift of -90° from the sine function.

Note the vertical lines are 90° apart in the animation.

A classic example of this effect is the Galilean moons of Jupiter. Their orbits are nearly circular with nearly uniform circular motion and they are seen approximately edge-on from the Earth. Hence on the sky, they oscillate approximately sinusoidally with amplitudes approximately equal to their mean orbital radii.

The mathematical relationship between uniform circular motion relates to sinusoidal motion has technological implications. The riprocating engine operating at constant rate converts the approximate sinusoidal motion (the linear motion of a piston in an engine cylinder) into the approximate uniform circular motion of a wheel of some kind.

Credit/Permission: User:LucasVB, 2014 / Public domain.
Image link: Wikipedia: File:Circle cos sin.gif.
Local file: local link: trig_sinusoid_animation.html.
File: Trigonometry file: trig_sinusoid_animation.html.