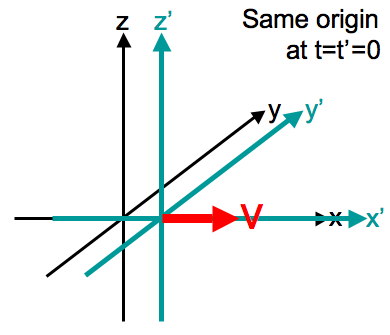
Caption: The standard image of a coordinate system for explaining both the Galilean transformations of classical physics and the Lorentz transformations of special relativity.

Features:

1. The primed coordinate system x-axis slides at velocity V along the x-axis of the unprimed coordinate system with the other axes being the same for both coordinate systems.

   At time zero, the 2 coordinate systems exactly overlapped.

2. The transformation formulae show how variable in one coordinate system are related to those in the other coordinate system.

   For example, the Galilean transformation for a velocity in the x direction from the unprimed to the primed coordinate system is

     v_x' = v_x - V   
   
     and the inverse is   
   
     v_x = v_x' + V  

   which is what you would expect.

3. The most striking fact about the Lorentz transformations is that the time coordinate changes between the coordinate systems.

   This does NOT happen with the Galilean transformations---which were the transformations that were thought to be exactly correct (by most people) before the discovery of special relativity in 1905 by Albert Einstein (1879--1955).