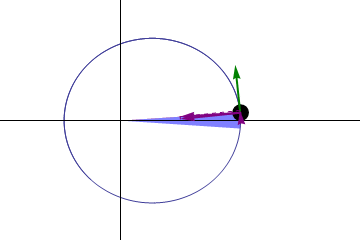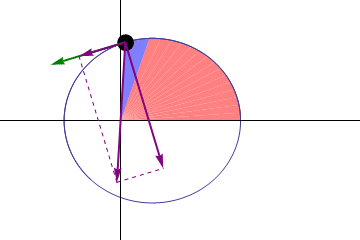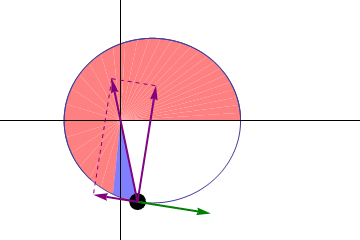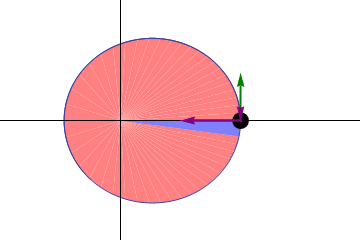
Caption: An animation of Kepler's 2nd law of planetary motion.

Features:

1. Kepler's 2nd law of planetary motion:
   The orbital radius of planet or other orbiting body acted on by a central force (which in general is NOT an inverse-square law force) sweeps out equal areas in equal times.

   This means the body moves faster the nearer it is to the center of force, fastest when closest, and slowest when farthest.

   Recall for astronomical orbits (which is usually what one means by orbits without qualification), closest approach is periapsis and farthest remove is apoapsis in general terminology.

2. In the animation, the green arrow represents the velocity of the body and the middle purple arrow represents central force on the body.

   The other two purple arrows represent the components of the central force in the direction of motion and perpendicular to the direction of motion.

3. Kepler's 2nd law is now understood to be a consequence of the conservation of angular momentum of an object acted on only by a central force (which in general is NOT an inverse-square law force).

   In a gravitationally-bound gavitational two-body system, gravity is the central force.

   Planets orbiting the Sun and moons orbiting a planet obey Kepler's 2nd law to high accuracy. Astronomical perturbations always forbid it from holding exactly.

4. Johannes Kepler (1571--1630) and immediately succeeding astronomers did use Kepler's 2nd law for calculating orbital motions by calculations by hand. Such calculations were very tedious, but gave far higher accuracy/precision than ever achieved before, and so they had to do them.

5. Among other things, Kepler's 2nd law broke the hold of uniform circular motion on the minds of astronomers. From Greco-Roman antiquity, astronomers had been fixated on the Platonic principle of uniform circular motion (probably NOT actually posited by Plato (428/427--348/347 BCE)) which held that the motions of the Heavens could only explained in terms of compounded uniform circular motions even though they had NO theory allowing them to predict how the compounding had to happen. They just fitted the observations with whatever compounded uniform circular motions worked well enough. Ptolemy (c.100--c.170 CE) actually violated the Platonic principle of uniform circular motion with his equant device without, however, denying the principle and later astronomers working in the tradition of ancient Greek astronomy considered this the crime of Ptolemy---including Nicolaus Copernicus (1473--1543).

   Platonic principle of uniform circular motion is, in fact, a WRONG theory of celestial mechanics.

   By dispensing with Platonic principle of uniform circular motion, Kepler helped to set path to the true dynamics found by Isaac Newton (1643--1727): i.e., Newtonian physics.

6. Note that Kepler by his Kepler's 1st law had dismissed epicycle models and put the Sun at the focus points of the elliptical orbits of all the planets including planet Earth in a heliocentric solar system model.