Image Questions
Which quantity is a vector?
What is this?
Is the top object at rest?
+ marks the center of mass and it can't be accelerated without ___________?
What laws of nature are being illustrated?
The black curve shows a parabolic trajectory for ballistic flight in the absence of ____________ and the other curves show quasi-parabolic trajectories with ____________.
What principle is being illustrated in this somewhat busy gif?
What is flowing through a complex system in this illustration?
Image Answers
Caption: Displacement and distance compared.
Displacement is a vector. It has a magntitude and a direction.
Credit: User:Stannered
Permission: Use under GNU Free Documentation License.
Image linked to Wikipedia.
Caption: "Sundial in thyme garden at Minnesota Landscape Arboretum. Photographed June 17, 2007 at 12:21 solar time (13:21 Daylight Savings Time)." I don't think this is really an antique sundial from 1642.
This is a horizontal sundial. Horizontal sundials are easy to read, but the markings cannot be evenly spaced in order to give the true solar time hours and the spacing is latitude dependent.
There are all kinds of sundials and they are rather complicated things because of all the geometry of angles, lines, and planes involved.
Credit: User:SEWilco.
Permission: Use under GNU Free Documentation License.
Image linked to Wikipedia.
Caption: Free body diagrams of objects: one on a horizontal plane; one one an inclined plane.
The top object could be moving with constant velocity in the horizontal direction.
Credit: User:Penubag.
Permission: Public domain at least in USA.
Image linked to Wikipedia.
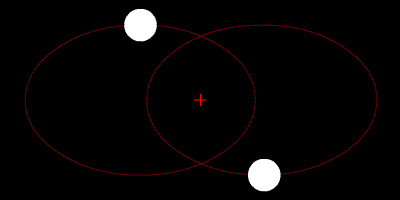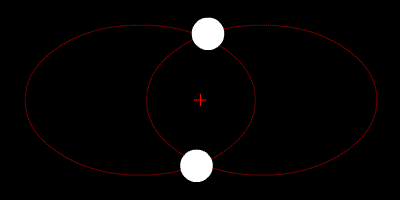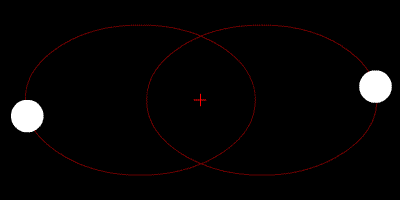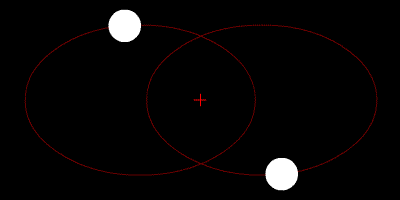
Caption: "Two bodies with similar mass orbiting around a common barycenter (red cross) with elliptic orbits." The barycenter is the center of mass.
ANS: a net external force---which isn't present here. The internal force of gravity, angular momentum, and classical mechanics keeps the equal-mass bodies orbiting their joint center of mass.
Credit: User:Zhatt.
Image linked to Wikipedia.
Permission: Public domain at least in USA.
Caption: The force of gravity between two spheres.
Gravity is always an attractive force, and so the two spheres attract each other.
Each sphere experiences a gravitational force equal in magnitude, but opposite in direction and so gravity is in agreement with Newton's 3rd law of motion.
No other forces are acting and the spheres start from rest.
Thus they move toward each other and are accelerating in accordance with Newton's 2nd law of motion
The larger sphere has more mass, and so has a smaller acceleration also in accordance with Newton's 2nd law of motion.
ANS: Newton's law of universal gravitation, Newton's 2nd law of motion, and Newton's 3rd law of motion.
Credit: User:Orion 8.
Permission: Use under GNU Free Documentation License.
Image linked to Wikipedia.
Caption: "Trajectories of three objects thrown at the same angle (70 degrees). The black object doesn't experience any form of drag and moves along a parabola. The blue object experiences Stokes' drag, and the green object Newton drag".
The curves with drag may not be for air or any other particular system.
ANS: drag AKA air resistance when the drag is for air.
Credit: User:AllenMcC. AKA Allen McCloud.
Image linked to Wikipedia.
Permission: Licensed under the Creative Commons Attribution ShareAlike 2.5.
Caption: "Elastic and Inelastic Collision. Example (m1 = 4kg, u1 = 5m/s, m2 = 4kg, u2=0m/s) of collision carts in perfectly inelastic collision showing the equations of momentum conserved and kinetic energy associated with some energy loss. This example (m1 = 4kg, u1 = 5m/s, m2 = 4kg, u2=0m/s) of collision carts in perfectly inelastic collision, notice the center of mass of the masses m1 and m2 is at the location of vcm = 2.5m/s always throughout the motion."
ANS: Conservation of momentum in the absence of an external net force. The two carts are collectively one system and the collision forces are internal forces.
Credit: User:Lookang.
Image linked to Wikipedia.
Permission: Licensed under the Creative Commons Attribution ShareAlike 2.5.
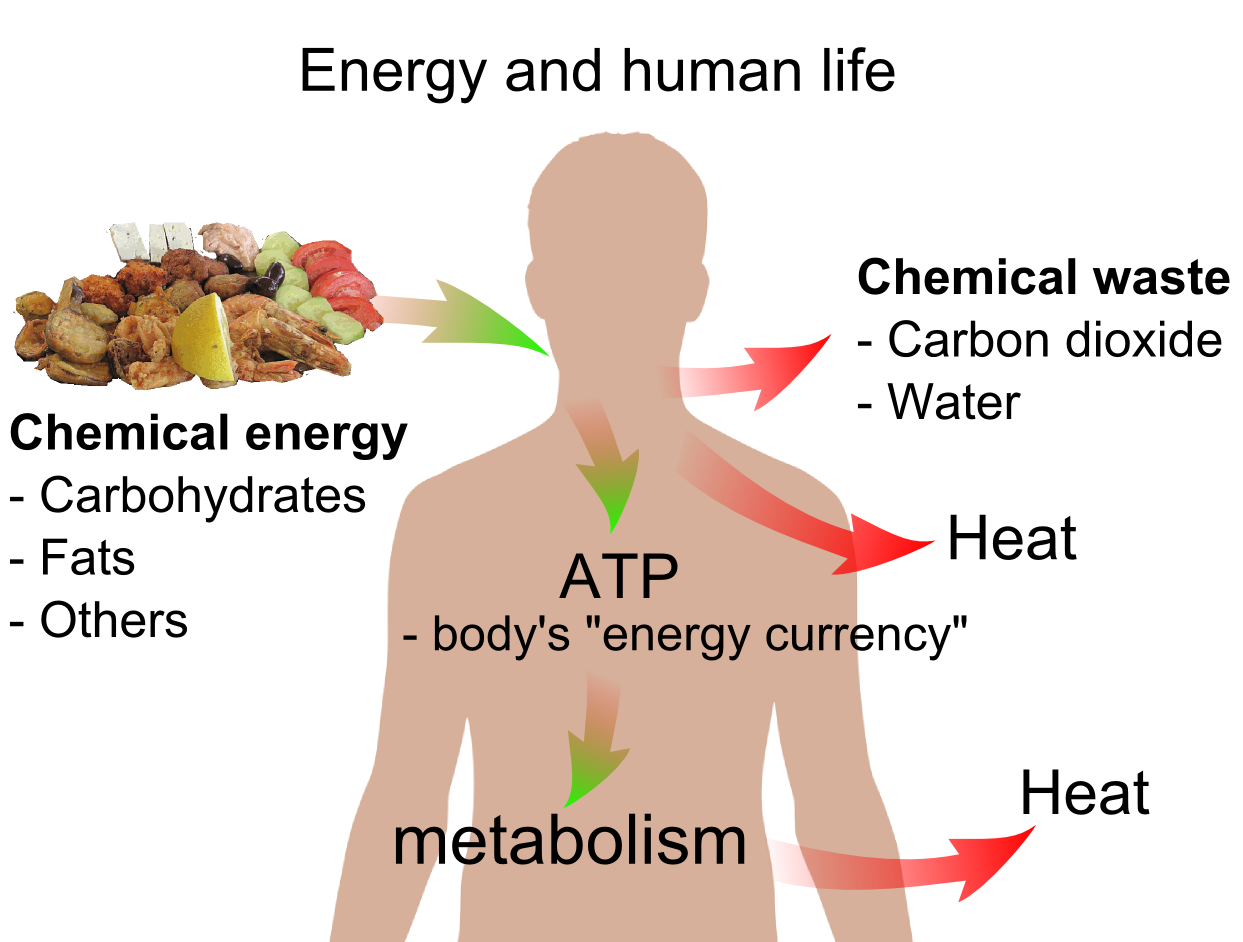
Caption: "Basic overview of energy and human life."
Immensely complex processes are going on in turning food energy (i.e., food chemical energy) into human chemical energy and then into human kinetic energy and then into waste heat (waste in the sense it is usually of no use to us) which ultimately flows out into space as the energy of infrared radiation.
In space, the infrared radiation will mostly cool off and dilute forever in our current favored version of Big Bang cosmology---which may not be correct.
But despite that complexity, we can crudely understand the processes in terms of energy flows and conservation of energy.
I think my own favored definition of energy helps to energy:
-
Energy is the conserved essence of structure
and transformability.
Credit: Mikael Haeggstroem.
Permission: Public domain at least in USA.
Image linked to Wikipedia.
Videos
-
Free Fall videos:
- Apollo 15 Hammer and Feather Experiment ``On the surface of the Moon at Hadley Rille, Apollo 15 Commander David Scott verifies Galileo's discovery that all objects in'' constant gravity fields fall with the same acceleration. Note gravity is weaker on the Moon and the free fall time is less.
- National Geographic Free Fallin' Felines A discussion of the high-rise syndrome. Cats like jumping out of high windows---they're just so smart. Longish, but OK for classroom.
-
Solar power videos:
- Solar Power 101 - how does sunlight turn into electricity Good. Not much on the physics. A commercial actually. Short enough for classroom.
- How PV cells produce electricity Not bad. Short enough for classroom.
- Solar Energy - The Future is Now Just a collage of solar power stations. But good to see.
- Solar Power in the US Southwest Good but a bit longish. All about concentrated solar power. A TV news item.
- Solar Photovoltaic Cells Part 1 Too long for classroom. All printed words and George Harrison for sound.
- Photovoltaics and the Photoelectric Effect Too long for classroom. Good for grade 6.
-
Nuclear bomb videos
- Tsar Bomba - Largest Nuclear Device Ever Tested (50MT) It's a lot like Dr. Strangelove (1964) for real.
- Atomic Bomb Footage Light heat flash before the shock wave arrives. Short enough for classroom.
- Christmas Island Atomic Bomb Test Short enough for classroom.
-
Orbit videos:
- Shoot a Cannonball into Orbit! Newton's cannonball animated. Good for the classroom.
- Halley's Comet Orbital Path Halley's comet does indeed have a clockwise orbit as viewed from above the NCP. Its orbit is a retrograde orbit. Short enough for classroom. Nice music.